Question
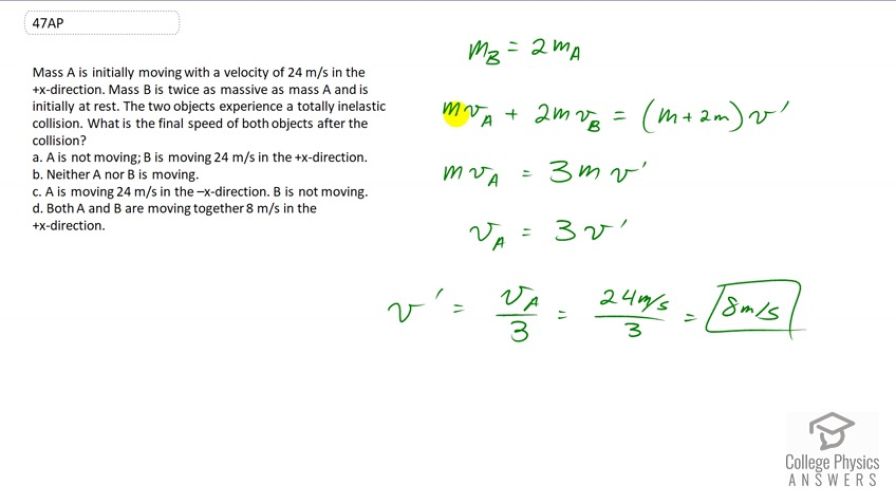
Mass A is initially moving with a velocity of 24 m/s in the +x-direction. Mass B is twice as massive as mass A and is initially at rest. The two objects experience a totally inelastic collision. What is the final speed of both objects after the collision?
- A is not moving; B is moving 24 m/s in the +x-direction.
- Neither A nor B is moving.
- A is moving 24 m/s in the –x-direction. B is not moving.
- Both A and B are moving together 8 m/s in the +x-direction.
Final Answer
(d)
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 47 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We have mass B is two times mass A, so when we write our conservation of momentum formula we can just write the letter m with no subscript because the first mass will be m and the second mass will be two m. So we have the total momentum initially is m vA plus two m times vB although this term is zero because mass B is initially at rest. Then after the collision the two objects stick together so there is a single thing of mass m plus two m and it has some speed v prime that we're going to find. So because this term became zero, this conservation of momentum equation reduces to m vA equals three m v prime. So we divide both sides by three m to solve for v prime. That means -- well, actually I guess it's just solve for -- I guess I did this in two steps didn't I? Well, it doesn't really matter. Yeah, so divide both sides by m and you get this and then divide both sides by three. You get v prime is vA over three. So that's 24 meters per second is the initial velocity of mass A and one third of that is eight meters per second. So the answer is D, they're both moving together eight meters per second in a positive direction because this is positive eight meters per second.