Question
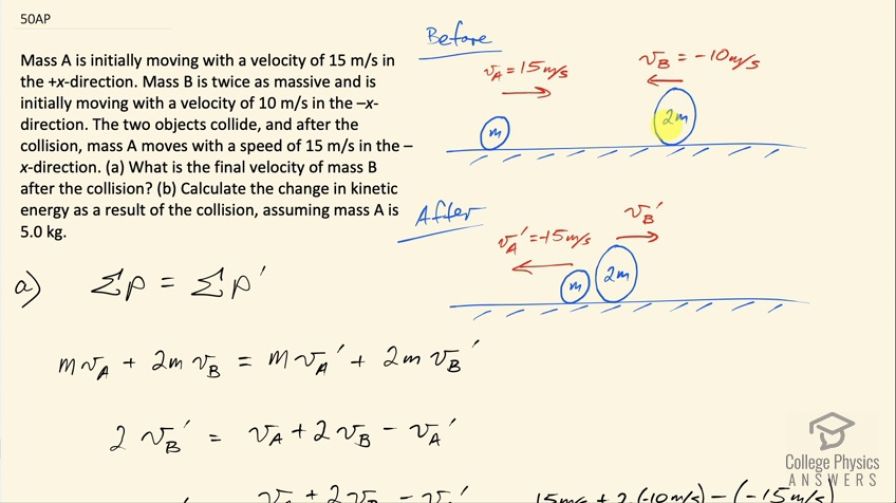
Mass A is initially moving with a velocity of 15 m/s in the +x-direction. Mass B is twice as massive and is initially moving with a velocity of 10 m/s in the –x-direction. The two objects collide, and after the collision, mass A moves with a speed of 15 m/s in the –x-direction. (a) What is the final velocity of mass B after the collision? (b) Calculate the change in kinetic energy as a result of the collision, assuming mass A is 5.0 kg.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 50 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Mass A is initially moving with a velocity of 15 meters per second to the right and mass B, which is a mass two times that of A is moving in the negative direction 10 meters per second. They collide and after collision, the object A is moving to the left with a speed of 15 meters per second and object B is moving to the right with some velocity that we don't know and we'll have to find so part (a) says find that velocity of mass B. So we know the total momentum before the collision equals the total momentum after collision and that is m times velocity A plus 2m times velocity B equals mass times velocity A after collision plus 2 times the m times velocity B after the collision. And we can divide both sides by m and also subtract v A prime from both sides and we have 2v B prime equals v A plus 2v B minus v A prime and then divide both sides by 2 to solve for v B prime. So that's v A plus 2v B minus v A prime all over 2. That's 15 meters per second plus 2 times negative 10 meters per second— the negative indicates that object B is moving to the left before the collision— and minus negative 15 meters per second so this minus a negative is an important detail— it will end up being plus there— and all that gets divided by 2 and we have positive 5 meters per second is the velocity of mass B after the collision. Part (b) asks us to find the change in kinetic energy so we take the total final kinetic energy and subtract from that the total initial kinetic energy. So that's one-half m times v A prime squared plus one-half times 2m because that's the mass of object B times object B's velocity after the collision squared and subtract from that one-half mv A squared plus one-half times 2mv B squared. So we factor out the m and then this one-half times 2 becomes the number 1. So we have just v B prime squared there and we have one-half v A prime squared minus v A squared minus v B squared and all this is multiplied by the common factor m— m we are told is 5.0 kilograms— and we multiply that by one-half times negative 15 meters per second squared plus 5 meters per second squared minus one-half times 15 meters per second squared minus negative 10 meters per second squared and this is negative 375 joules. So 375 joules of kinetic energy is lost due to the collision.
