Question
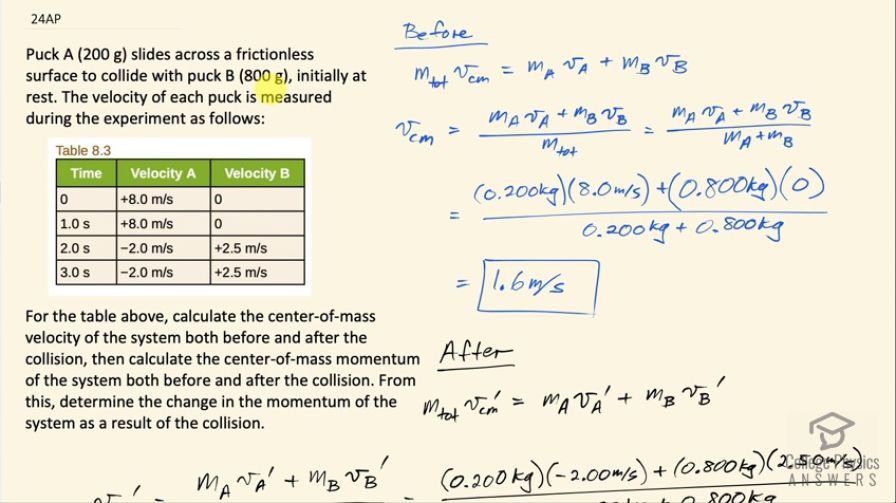
For the table 8.3 below, calculate the center-of-mass velocity of the system both before and after the collision, then calculate the center-of-mass momentum of the system both before and after the collision. From this, determine the change in the momentum of the system as a result of the collision.
Final Answer
The center of mass velocity is both before and after collision. The total momentum before and after collision is . The change in momentum after the collision is zero.
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 24 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Puck A of mass 200 grams is sliding across a frictionless surface to collide with puck B that has a mass of 800 grams and it's initially at rest. The velocity of each puck is measured... shown in this data table so at time 0, puck A is moving at 8.0 meters per second and at 1.0 second, it's still 8.0 meters per second and puck B is zero in both cases and then there's a collision and at 2.0 seconds, puck A has rebounded going in the opposite direction now at 2.0 meters per second whereas puck B is moving in the puck A's original direction— the positive direction—at a speed of 2.5 meters per second and they carry on at a constant speed. So the question is calculate the center of mass velocity of the system both before and after collision and then find the center of mass momentum both before and after collision and then find the change in momentum of the system as a result of the collision although we'll find that there is no change in momentum because momentum is conserved. So before the collision, we can say that the total mass multiplied by the velocity of the center of mass is equal to the total momentum of each component in the system and so we can solve for the center of mass velocity by dividing both sides by the total mass and so the center of mass velocity then is m Av A plus m Bv B divided by m A plus m B and then plugging in numbers, we have 0.200 kilograms times 8.0 meters per second plus 0.800 kilograms times 0 because initially puck B is not moving at all divided by 0.200 plus 0.800 and that's 1.6 meters per second. After the collision, we have the total mass times the velocity of the center of mass after collision and the after is indicated by a prime equals the momentum of puck A after collision plus momentum of puck B after collision and we'll divide both sides by the total mass where I have written m total here as m A plus m B and we have 0.200 kilograms times negative 2.00 meters per second— the velocity of puck A is negative 2.00 meters per second there after collision— and plus 0.800 kilograms times 2.50 meters per second divided by the total mass and that is still positive 1.6 meters per second. So the change in momentum is the total momentum afterwards minus the total momentum before collision and that's m total times v cm prime minus m total times v cm the total mass of course is the same before and after collision and we have seen that the velocities of the center of mass are the same before and after collision—both 1.6— and so this total change in momentum will be zero. I guess we are supposed to calculate the center of mass momentum of the system before and after collision and so let's do that then too. So the momentum of the center mass will be this total mass m A plus m B times the velocity of the center of mass which is... you can use v cm prime or v cm because they are the same in either case and so we have the momentum after the collision of the center of mass equals the momentum before the collision and this works out to 0.200 kilograms plus 0.800 kilograms times 1.6 meters per second and calculating that well, that's gonna be 1.6 because 0.200 plus 0.800 is 1— that makes an easy calculation— and that's 1.6 kilogram meters per second.
