Question
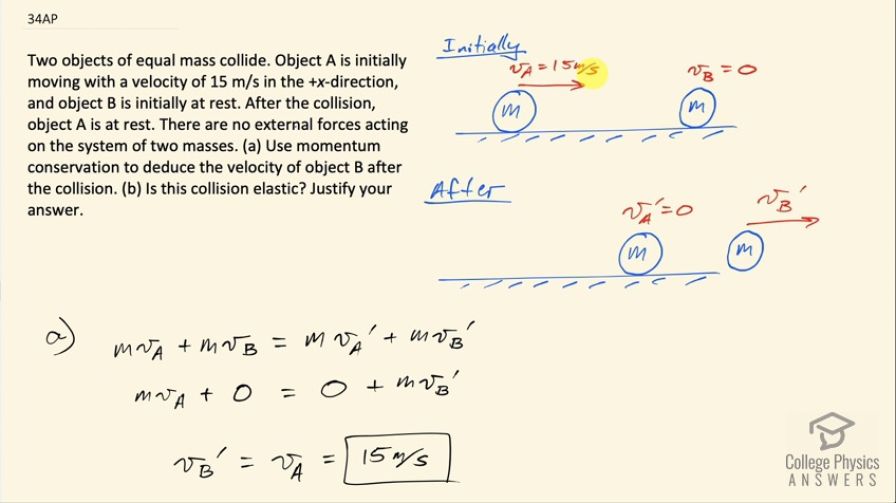
Two objects of equal mass collide. Object A is initially moving with a velocity of 15 m/s in the +x-direction, and object B is initially at rest. After the collision, object A is at rest. There are no external forces acting on the system of two masses. (a) Use momentum conservation to deduce the velocity of object B after the collision. (b) Is this collision elastic? Justify your answer.
Final Answer
- Yes, the collision is elastic since kinetic energy is conserved.
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 34 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. Two objects of equal mass collide: object A has an initial velocity of 15 meters per second and object B is initially at rest. After the collision, object A is at rest and then object B is moving with some velocity that we have to find and this velocity after collision is indicated with a prime symbol. Okay! So in part (a), we'll use momentum conservation to figure out what velocity B prime is. We know that the total momentum initially equals the total momentum after collision and so we have mass times velocity A plus zero since velocity B is initially zero and then that's going to equal zero since that's what velocity A prime is after collision plus mass times v B prime. We can divide both sides by m and then we solve for v B prime is the same as v A in other words, positive 15 meters per second. In part (b), we are going to figure out whether or not this collision is elastic? So that would mean that the total initial kinetic energy is the same as the total final kinetic energy; if that's true then we can say yes, the collision is elastic that's the meaning of elastic collisions is that kinetic energy is conserved. So we have one-half mass times velocity A squared plus one-half mass times velocity B squared all before collision equals one-half mass times velocity A after collision squared plus one-half mass times velocity B after the collision squared. So that's one-half times v A squared plus zero equals zero plus one-half v B prime squared where I have divided both sides by m and then also substituted zero in for v B and v A prime and we have 15 meters per second is the initial velocity of mass A and we square that, divide by 2 and ask whether or not that equals the velocity of mass B after the collision, which is 15 squared divided by 2 and sure these sides are equal so yes, the collision is elastic.