Question
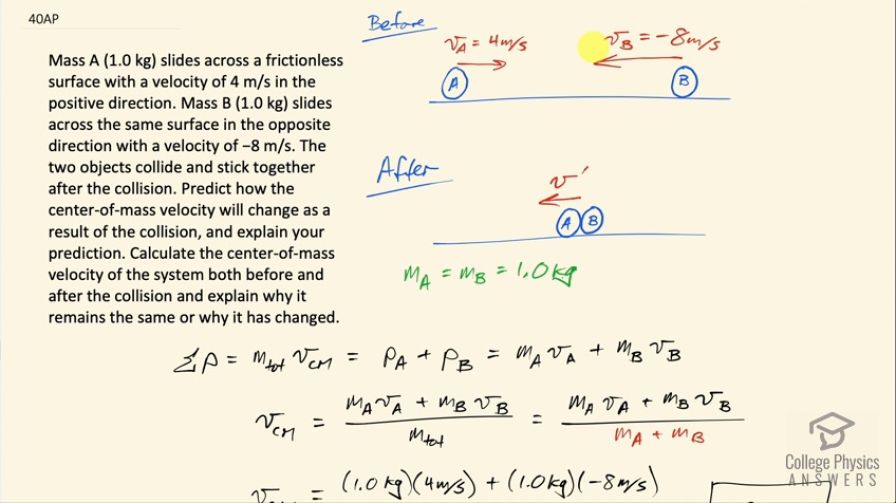
Mass A (1.0 kg) slides across a frictionless surface with a velocity of 4 m/s in the positive direction. Mass B (1.0 kg) slides across the same surface in the opposite direction with a velocity of −8 m/s. The two objects collide and stick together after the collision. Predict how the center-of-mass velocity will change as a result of the collision, and explain your prediction. Calculate the center-of-mass velocity of the system both before and after the collision and explain why it remains the same or why it has changed.
Question by OpenStax is licensed under CC BY 4.0
Final Answer

vCM=−2 m/sv_\textrm{CM} = -2 \textrm{ m/s}
vCM′=−2 m/sv_\textrm{CM}' = -2 \textrm{ m/s}
Since vCM=ptotmtotv_\textrm{CM} = \dfrac{p_\textrm{tot}}{m_\textrm{tot}}, vCMv_\textrm{CM} can't change after the collision since neither ptotp_\textrm{tot}, nor mtotm_\textrm{tot} change.

Solution video

OpenStax College Physics for AP® Courses, Chapter 8, Problem 40 (Test Prep for AP® Courses)

OpenStax College Physics, Chapter 8, Problem 40 (AP) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 8, Problem 40 (AP) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. So mass A moves to the right with a speed of 4 meters per second and whereas mass B is moving to the left with a velocity of negative 8 meters per second; they collide and then stick together and so they move with some common velocity we'll call v prime and we can tell since the masses are the same with B having a greater magnitude velocity that its initial direction will be the direction of the combined mass after colliding so it's a bit to the left here. Mass A and mass B are both 1.0 kilogram so we have to figure out what the velocity of the center of mass of the system is before and after the collision and we'll see whether or not that center of mass velocity changes. So the sum of the momentum before the collision equals the total mass times the center of mass velocity before the collision and so that's the momentum of object A plus momentum of object B so that's m A times initial velocity A plus mass B times initial velocity B and we can solve this for the center of mass velocity by dividing both sides by the total mass and then substitute mass A plus mass B in place of total mass. So that's 1.0 kilogram times 4 meters per second plus 1.0 kilogram times negative 8 meters per second divided by 1.0 kilogram plus 1.0 kilogram and that's negative 2 meters per second is the velocity of the center of mass of the system. Now since momentum is conserved, we can figure out what the velocity of this combined object is— v prime—after the collision and so this is the total momentum before collision and it equals the single object of mass m A plus m B stuck together times its common velocity v prime and this also is the total mass times the center of mass velocity after the collision and so we can solve for this v CM prime by dividing both sides by this total mass and then we have this expression here, which is the same as the expression we had before for the center of mass velocity before the collision and so the center of mass velocity after the collision is the same as the center of mass velocity before collision and that is negative 2 meters per second— no calculation necessary here. So the center of mass velocity can't change because it equals the total momentum divided by the total mass and neither of these things are changing as a result of the collision because momentum is conserved—this total momentum doesn't change— and the mass is not changing either so the center of mass velocity can't change in a collision where momentum is conserved.