Question
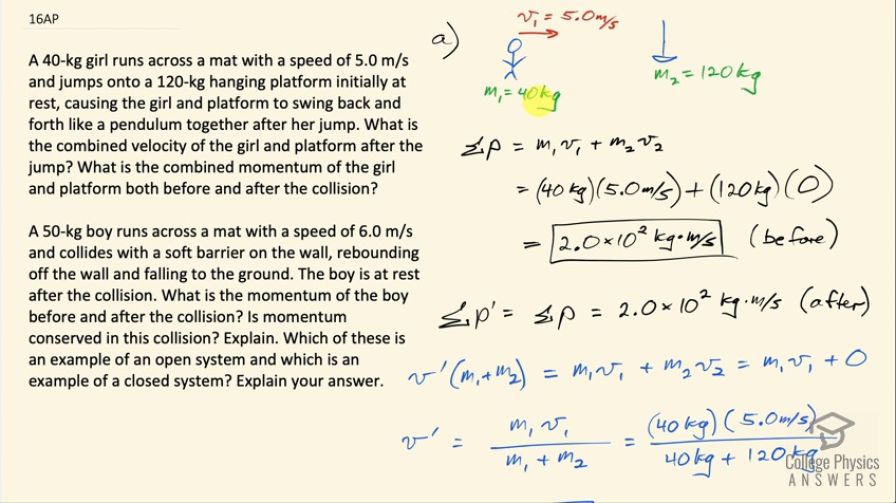
A 40-kg girl runs across a mat with a speed of 5.0 m/s and jumps onto a 120-kg hanging platform initially at rest, causing the girl and platform to swing back and forth like a pendulum together after her jump. What is the combined velocity of the girl and platform after the jump? What is the combined momentum of the girl and platform both before and after the collision?
Final Answer
- This is a closed system. The combined momentum of the girl and platform both before and after collision is
- This is an open system. The total momentum before collision is . The total momentum after collision is
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 16 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A girl is running at 5.0 meters per second towards a hanging platform, the mass of the girl is 40 kilograms and the platform mass is 120 kilograms and the platform is initially at rest and so the girl is going to jump onto the platform and then they will move off together as a single combined mass thing and our job is to figure out what is the speed of that inelastic collision or the speed of this object consisting of the girl stuck to the platform. So the initial momentum is in total the mass of the girl times her velocity plus the mass of the platform multiplied by its velocity. So that's 40 kilograms times 5.0 meters per second plus the platform mass times zero because it's not moving initially and so initially, the total momentum of the system is 2.0 times 10 to the 2 kilogram meters per second. The total momentum after collision, which is indicated by this prime here is going to equal the total momentum initially because momentum will be conserved— this is a closed system, there is no external object exerting a net force on our system; all this momentum is horizontal so we can ignore gravity which is perpendicular to that— and so the total momentum after collision is the same as before— 2.0 times 10 to the 2 kilograms meters per second— and the total momentum after collision is the velocity that the two have together we'll call that v prime multiplied by the combined mass m 1 plus m 2 once the girl is on the platform and all of that equals the initial momentum, which is mass of the girl times her initial velocity because the platform has zero momentum initially and then we divide both sides by this total mass—m 1 plus m 2— and then we get v prime then is m 1v 1 over m 1 plus m 2. So that's 40 kilograms times 5.0 meters per second divided by 40 kilograms plus 120 kilograms and that is 1.3 meters per second. So the girl and platform are a closed system and all changes in momentum are accounted for, there's no external thing exerting a net force in the direction of the momentum here. Okay! And then part (b) of this question talks about a boy hitting a padded wall and that is an open system because we are not accounting for all the momentum changes; there's going to be a momentum change of the wall but it's not accounted for in this problem and so the problem is not closed, it's not including everything it needs to include for conservation of momentum. So the total initial momentum is the mass of the boy, which is 50 kilograms times the boy's initial speed of 6.0 meters per second which is 300 kilograms meters per second and then total momentum after is the mass of the boy times the boy's velocity after collision and that's 50 kilograms times 0 because they come to a rest and so momentum is not conserved in this open system because we haven't included all the things. If we were to broaden the system to include the wall and the Earth then momentum would be conserved but it's not, practically speaking, possible to measure the momentum change of the Earth because it would be so negligibly small. Well I mean the momentum change would be the same as that of the boy, what would be negligibly small is the Earth's velocity... recoil velocity... that would be practically impossible to measure.
