Question
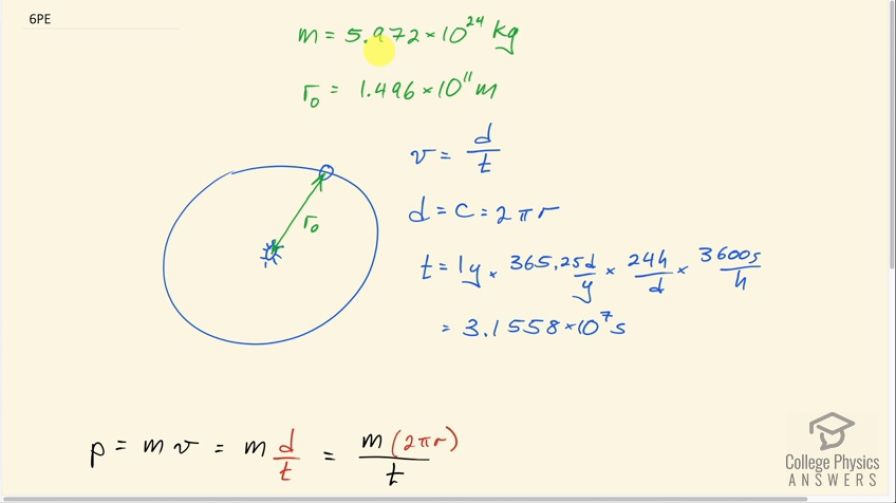
The mass of Earth is and its orbital radius is an average of . Calculate its linear momentum.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 6 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
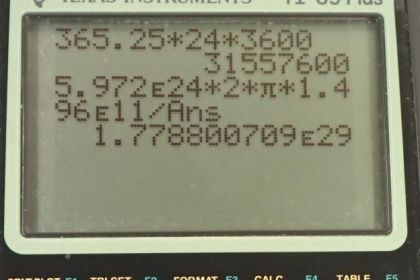
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to calculate the linear momentum of the Earth as it revolves around the Sun. Its mass is 5.972 times 10 to the 24 kilograms and we are given the orbital radius on average of its orbit— 1.496 times 10 to the 11 meters. What we want to know is the speed of the Earth and that's gonna be the distance that it travels divided by the time it takes to do that and we know the time it takes to go one full revolution—that's 1 year— and so we will find the distance of one revolution which is the circumference of this circle which has a formula 2πr and then we are gonna divide that distance by this time of one year expressed in seconds by multiplying by 365.25 days per year times 24 hours per day times 3600 seconds per hour. And so we have momentum of the Earth then is its mass times its speed, which is distance over time— distance being 2πr— and then we substitute in all the numbers that we have above here and we end up with 1.78 times 10 to the 29 kilogram meters per second.