Question
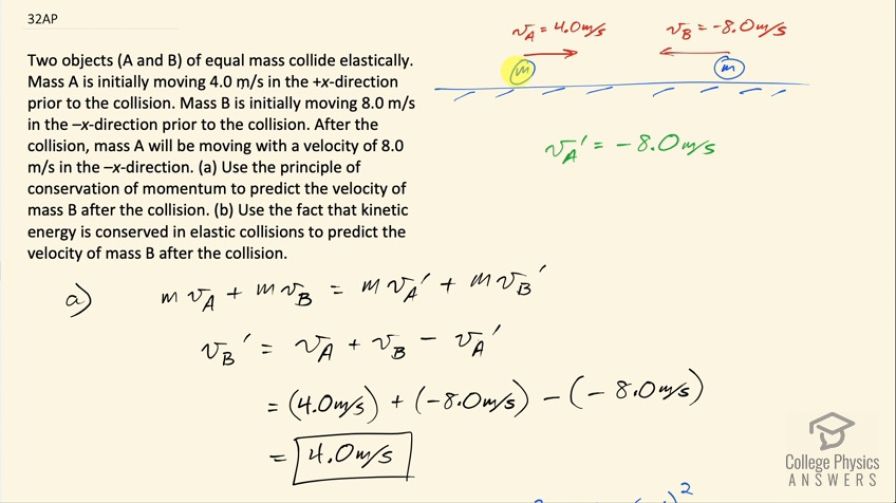
Two objects (A and B) of equal mass collide elastically. Mass A is initially moving 4.0 m/s in the +x-direction prior to the collision. Mass B is initially moving 8.0 m/s in the –x-direction prior to the collision. After the collision, mass A will be moving with a velocity of 8.0 m/s in the –x-direction. (a) Use the principle of conservation of momentum to predict the velocity of mass B after the collision. (b) Use the fact that kinetic energy is conserved in elastic collisions to predict the velocity of mass B after the collision.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 32 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We have two objects of equal mass approaching each other: mass A has an initial velocity before collision of 4.0 meters per second to the right and mass B has an initial velocity of negative 8.0 meters per second and it's moving to the left. After the elastic collision— elastic meaning that the kinetic energy is conserved during the collision— we are told what the velocity of mass A will be and we are gonna indicate that this is the velocity of A after collision with this prime symbol and so after colliding, mass A will be moving with a velocity of negative 8.0 meters per second. So in part (a), we are meant to use the conservation of momentum principle to figure out what is the mass or the velocity of mass B after the collision. So the total momentum initially has to equal the total momentum after collision and the m's don't need prime's since the masses are not changing but the velocities are so after collision, we have v A prime here and we have v B prime here so we are taking the product of mass and velocity for each object before and after collision and we are gonna solve for v B prime. So we'll divide everything by m and then subtract v A from both sides and so we have v B prime or sorry... v A prime is getting subtracted from both sides and then we are isolating v B prime so v B prime is v A plus v B minus v A prime. So that's 4.0 meters per second— initial velocity of mass A— plus negative 8.0 meters per second— initial velocity of mass B— minus the negative velocity of object A after collision and this is 4.0 meters per second and it's positive so mass B will be moving to the right. Basically they are exchanging velocities, which is what we expect in an elastic collision with objects of equal mass. Part (b) says get the same answer but use conservation of kinetic energy instead of conservation of momentum. So we have one-half mass times velocity A squared plus one-half mass times velocity B squared— this is the total kinetic energy before collision— has to equal the total kinetic energy after collision one-half mass times v A prime squared plus one-half mass times v B prime squared and we divide both sides by one-half times m and then we have v A squared plus v B squared equals v A prime squared plus v B prime squared and solving for v B prime squared, we subtract v A prime squared from both sides and then switch the sides around and v B prime squared equals v A squared plus v B squared minus v A prime squared. Then we take the square root of both sides and we get v B prime equals the square root of v A squared plus v B squared minus v A prime squared. Now strictly speaking, we can't be sure just from the mathematics whether this is a positive or a negative; when you take the square root of both sides of an equation, you can always consider both possibilities but we can tell from the Physics here that the object B is gonna be moving to the right if object A is moving so fast to the left. So in a sense, we are kinda using our knowledge of conservation of momentum anyhow because strictly looking only at conservation of energy leaves us with an ambiguous result in that this velocity can either be positive or negative but anyway, we are gonna take the positive result here based on our knowledge of the conservation of momentum. Okay! So that's a square root of 4.0 meters per second— velocity of object A initially—squared plus negative 8.0 meters per second squared minus negative 8.0 meters per second squared and we end up with the square root of 16.0, which is 4.0 meters per second.