Question
The ceramic glaze on a red-orange Fiestaware plate is and contains 50.0 grams of , but very little . (a) What is the activity of the plate? (b) Calculate the total energy that will be released by the decay. (c) If energy is worth 12.0 cents per , what is the monetary value of the energy emitted? (These plates went out of production some 30 years ago, but are still available as collectibles.)
Final Answer
Solution video
OpenStax College Physics, Chapter 31, Problem 62 (Problems & Exercises)
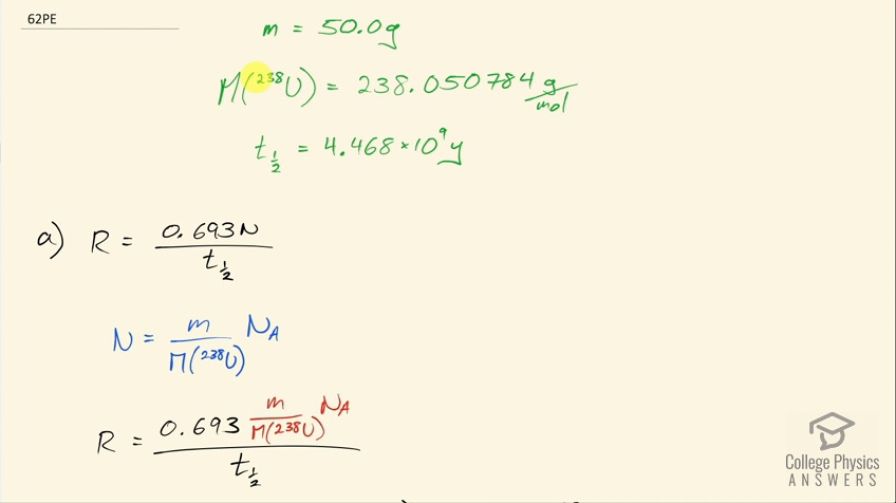
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
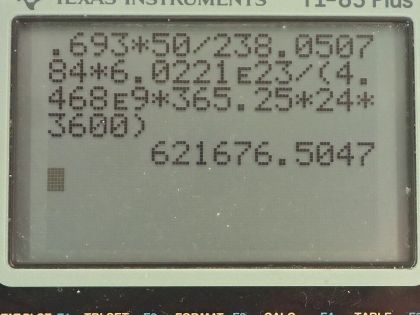
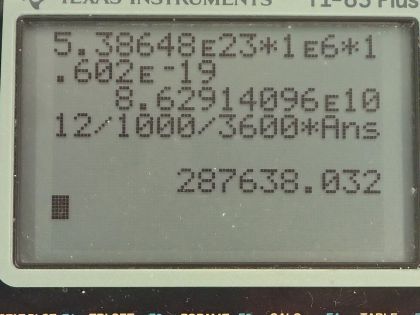
This is College Physics Answers with Shaun Dychko. An old Fiesta Ware dinner plate contains 50.0 grams of uranium-238 and the molar mass of uranium-238 is 238.050784 grams per mol and its half-life is 4.468 times 10 to the 9 years and I looked these up in the appendix at the end of the textbook. Question (a) is asking for the activity of the plate? So the activity is 0.693 times the number of atoms of uranium-238 divided by the half-life and the number of atoms we can figure out based on the mass and divide that mass by the molar mass and then multiply by Avogadro's number and that will give us the number of atoms. So this is 0.693 times 50.0 grams divided by 238.050784 grams per mol times Avogadro's number giving us the total number of atoms in the numerator here and then we divide that by the half-life and we convert the number of years into seconds and we have 6.22 times 10 to the 5 becquerels so that's 6.22 times 10 to the 5 decays per second, in other words. Part (b) is asking to calculate the total energy that will be released after all these atoms decay. So the first thing we need to do is figure out how much energy is released per decay and there are two different decay pathways for uranium-238. On the one hand, it does a gamma decay and on the other hand, it does I think an alpha decay here. So 77 percent of the decays release 4.27 megaelectron volts and then 23 percent decays release 4.22 megaelectron volts so we'll take a weighted average of those two to get the energy per decay. So that's 4.22 times 23 percent plus 4.27 times 77 percent and we will take 4.2585 megaelectron volts per decay. So the total energy released then is the total number of atoms multiplied by the energy per decay on average and so that's 50.0 grams divided by the molar mass times Avogadro's number times this energy per decay and then convert the megaelectron volts into joules by multiplying by 1 times 10 to the 6 electron volts per megaelectron volt and then by 1.602 times 10 to the minus 19 joules per electron volt and we have 8.63 times 10 to the 10 joules. Now if energy is worth 12 cents per kilowatt-hour, what is the financial value of the energy emitted? So the total value or the cost if you were to buy this this electricity produced by this power output or energy output I should say is 12.0 cents per kilowatt-hour and then we multiply by 1 kilowatt for every 1000 watts and then by 1 hour for every 3600 seconds and now this denominator has units of watt-seconds and a watt is joule per second so when we multiply the joules per second by seconds, we end up with joules so this denominator is joules. Then we multiply that by 8.6291 times 10 to the 10 joules of energy released in the decay and these joules cancel with these joules and we are left with our answer in cents. So that's... (what is that) 287638 cents, which we convert into dollars by dividing by 100 and that's 2880 dollars.