Question
The Galileo space probe was launched on its long journey past several planets in 1989, with an ultimate goal of Jupiter.
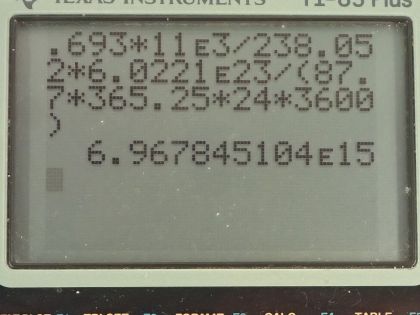
Its power source is 11.0 kg of , a by-product of
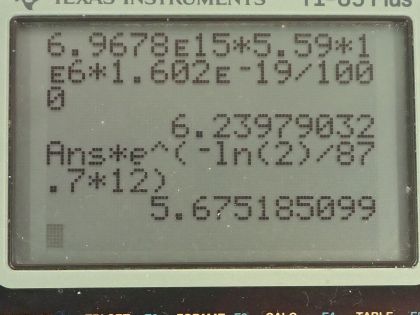
nuclear weapons plutonium production. Electrical energy is generated thermoelectrically from the heat produced when the 5.59-MeV particles emitted in each decay crash to a halt inside the plutonium and its shielding. The half-life of is 87.7 years. (a) What was the original activity of the in becquerel? (b) What power was emitted in
kilowatts? (c) What power was emitted 12.0 y after launch? You may neglect any extra energy from daughter nuclides and any losses from escaping rays.
Final Answer
Solution video
OpenStax College Physics, Chapter 31, Problem 64 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The Galileo space probe was launched toward Jupiter in 1989 and it had 11.0 kilograms of plutonium-238 on it to generate electricity and we are asked to figure out what is the original activity of this plutonium-238 when it was launched? So the energy per decay we are told is 5.59 megaelectron volts per decay and the half-life is 87.7 years and we convert the kilograms into grams and the molar mass of plutonium... well, I look up plutonium in the appendix and there's only plutonium-239 listed there so we have 239.052 atomic mass units or grams per mol I guess is what we are working with here actually... grams per mol... and I am going to subtract away 1.000 gram per mol which is approximately what we would expect then for plutonium-238—1.000 less than whatever the mass is for plutonium-239— because each nucleon has approximately a mass of 1.000 gram per mol at least to four decimal places I think it does. So this is our calculated mass for plutonium-238. So the activity then is 0.693 times the number of atoms of plutonium-238 divided by the half-life and the number of atoms is the mass divided by the molar mass times Avogadro's number so we substitute that in place of N and so the activity then is 0.693 times 11.0 times 10 to the 3 grams divided by 238.052 grams per mol times 6.0221 times 10 to the 23 atoms per mol and divide by the half-life converted into seconds and we end up with 6.97 times 10 to the 15 becquerels. Part (b) is asking what power was emitted in kilowatts? So the power at this original time when it was first launched is the activity or the decays per second multiplied by the energy per decay and then we convert this into kilowatts by multiplying the megaelectron volts by 1 times 10 to the 6 electron volts per megaelectron volt and then convert that into joules by multiplying by 1.602 times 10 to the minus 19 joules per electron volt and this will give us an answer in joules per second, which is watts and then multiply by 1 kilowatt for every 1000 watts and we end up with 6.24 kilowatts. Part (c) is asking what is the power 12.0 years after the space probe was launched? So we know that activity at some time equals some original activity multiplied by e to the negative λt, where λ is some decay constant which is natural logarithm of 2 divided by half-life but first let's express this activity as power because we can multiply both sides by energy per decay and then that gives us energy per time because this is decay per time or decays per second and then we are multiplying by energy per decay so that gives us energy per second, which is power. So the power after sometime then is the original power times e to the negative λt. So then we plug in this expression for λ here and we have 6.2398 kilowatts— original power— times e to the negative of natural logarithm of 2 divided by 87.7 years—half-life— times 12.0 years after launch and that's 5.68 kilowatts.