Question
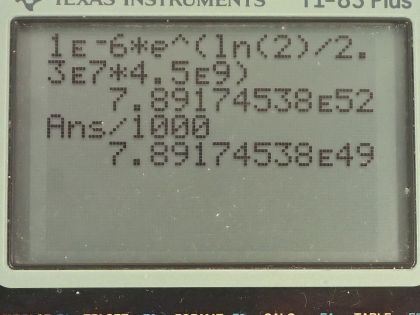
A nuclear physicist find of in a piece of uranium ore and assumes it is primordial since its half-life is . (a) Calculate the amount of that would had to have been on Earth when it formed ago for to be left today. (b) What is unreasonable about this result? (c) What assumption is responsible?
Final Answer
- This is many orders of magnitude greater than the mass of the entire Earth,
- Either the material is not , or it has recently been created in a nuclear reactor.
Solution video
OpenStax College Physics, Chapter 31, Problem 66 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A nuclear physicist discovers 1.0 microgram of uranium-236, which has a half-life of 2.3 times 10 to the 7 years and a molar mass of 236.045562 grams per mol, which I looked up in the appendix at the end of the textbook and the question is calculate the amount of uranium-236 that would have existed when the Earth was formed 4.5 billion years ago in order for there to be only 1.0 microgram left? So this is working on the assumption that the source of this uranium-236 is the primordial formation of the Earth so long ago. Now we know that the number of atoms left after sometime will be the original number of atoms times e to the negative of this decay constant λ times t but then we'll multiply both sides by e to the positive λt and then we end up getting the original number of atoms is this current number of atoms times e to the positive λt. Now the number of atoms is the mass divided by the molar mass times Avogadro's number and you can say the same about the original number of atoms in terms of the original mass and we can substitute for each of these and we are developing a different formula here for mass because now we can divide both sides by Avogadro's number and multiply both sides by molar mass and we end up with having the original mass is the current mass times e to the λt where I have done a substitution for λ and turned it into natural logarithm of 2 divided by half-life. So the original mass then was 1.0 times 10 to the minus 6 grams times e to the natural logarithm of 2 divided by 2.3 times 10 to the 7 years— that's the half-life there— times 4.5 times 10 to the 9 years— which was when the Earth was formed— and this works out to 7.9 times 10 to the 49 kilograms after you convert the grams into kilograms here. That is way too massive! That's many orders of magnitude greater than the mass of the entire Earth, which is only 6.0 times 10 to the 24 kilograms. So this answer is unreasonable and so it means either the material is not uranium-236 after all or it was recently created in a nuclear reactor instead of created when the Earth was formed.