Question
(a) Write the complete decay equation for . (b) Find the energy released in the decay.
Final Answer
- Please see the solution video.
Solution video
OpenStax College Physics, Chapter 31, Problem 36 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
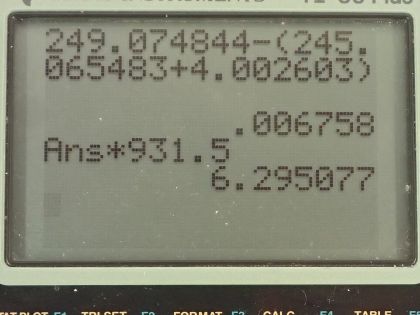
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We need to write the complete α decay equation for californium-249; alpha decay means that one of the daughter nuclides will be a helium nucleus which has 2 protons and 2 neutrons for a total of 4 nucleons. Now californium if you look it up in the appendix in the text book, it has a atomic number of 98 so that means it has 98 protons so we write 98 here and with 2 protons being used up for the helium nuclide that leaves 96 protons left over for this unknown thing which we now know what it is because its atomic number is 96 and it is curium and the mass number for this nuclide will be 249 total number of nucleons minus the 4 used up for the helium nuclide leaving 245 left over. So here's the full equation with curium written as curium-245. Okay! The next question is how much energy is produced in this decay? So that will equal the mass defect times c squared where the mass defect is the word to describe how much mass is missing in these daughter nuclides compared with what we started with in the parent nuclide. So the mass difference is the atomic mass of californium-249 minus the total atomic masses of a helium atom and a curium atom and when I say atom, I am referring to an atom that has the electron surrounding the nucleus and because there are no electrons being produced or destroyed in this equation, we have an equal number of electrons in the californium atomic mass as we do in the total atomic masses of curium and helium and so they subtract out and we don't need to worry about the electron masses because what we are after is just the difference in masses of these nuclides. Okay! So when we look up californium in our data table, we get this atomic mass for it and then we get these other atomic masses for curium and helium and we are left with the difference of 0.006798 atomic mass units which we then multiply by 931.5 megaelectron volts per c squared for every atomic mass unit and then multiply that by c squared and then we end up with 6.295 megaelectron volts.