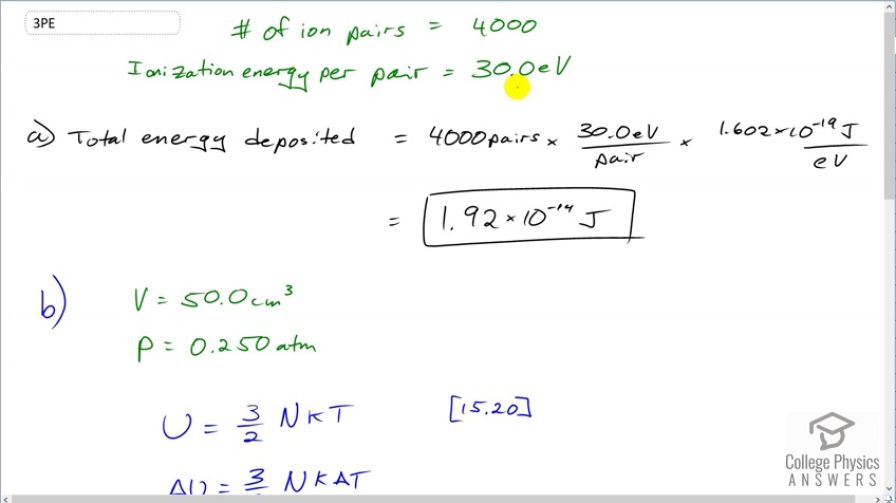
Question
(a) A particle of ionizing radiation creates 4000 ion pairs in the gas inside a Geiger tube as it passes through. What minimum energy was deposited, if 30.0 eV is required to create each ion pair? Convert the energy to joules or calories. (b) If all of this energy is converted to thermal energy in the gas, what is its temperature increase, assuming 50.0 cm350.0 \textrm{ cm}^3 of ideal gas at 0.250-atm pressure? (The small answer is consistent with the fact that the energy is large on a quantum mechanical scale but small on a macroscopic scale.)
Question by OpenStax is licensed under CC BY 4.0
Final Answer
  1. 1.92×10−14 J1.92\times 10^{-14}\textrm{ J}
  2. 2.96×10−12C∘2.96\times 10^{-12}\textrm{C}^\circ

Solution video

OpenStax College Physics, Chapter 31, Problem 3 (Problems & Exercises)

OpenStax College Physics, Chapter 31, Problem 3 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 31, Problem 3 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. 4000 ion-pairs were created in the gas in the Geiger counter. And we know that the ionization energy per pair is 30 electron volts and so question (a) asks us, what total energy must have been deposited in the gas in order to create 4000 pairs. So we have 4000 pairs multiplied by 30 electron volts required per pair and then times by 1.602 times 10 to the minus 19 joules per electron volt giving us 1.92 times 10 to the minus 14 joules of energy. Now part (b) asks us, assuming we have 50 cubic centimeters of gas at an atmospheric pressure of 0.250 atmospheres and it's an ideal gas, what temperature increase will it experience as a result of the energy that was deposited in the gas? Well in chapter 15, equation 20, we know that the total internal energy of an ideal gas is 3 over 2 times the number of gas particles times Boltzmann's constant times its temperature and we can figure out the change in the internal energy which is this energy that's deposited is gonna be 3 over 2 Nk times the change in temperature and we can solve for this change in temperature by multiplying everything by 2 over 3 Nk on both sides and then switching the sides around. So we have change in temperature is 2 times the change in internal energy or the energy deposited in other words, divided by 3 times the number of particles times Boltzmann's constant. Now we don't know what this is but we can replace it with things that we do know using the ideal gas law which says that the pressure times the volume of a gas is the number of particles times Boltzmann's constant times the temperature and we can rearrange this to solve for 1 over Nk by multiplying everything by 1 over NkPV and the PV cancels on the left leaving us with 1 over Nk and on the right-hand side, we have the Nk canceling leaving us with T over PV and the reason this is useful is because this contains the factor 1 over Nk here and we'll replace it then with all of this and that's what we do in the next line. So we have change in temperature is 2 times T from this times ΔU over 3PV; this all replaced 1 over Nk here. OK. And then we plug in the numbers that we know. So we have 2 times an assumed temperature of 20 degree Celcius which we have to convert into Kelvin by adding 273.15 multiplied by the energy deposited by this ionizing particle and divide that by 3 times the pressure which we have to express in units of Pascals and then multiply that by the volume which has to be in units of cubic meters because we always need meters and kilograms and seconds—mks units— in all of our questions; pascals is an mks unit. This works out to 2.96 times 10 to the minus 12 Celcius degrees is the change in temperature.