Question
A 2.00-T magnetic field is applied perpendicular to the path of charged particles in a bubble chamber. What is the radius of curvature of the path of a 10 MeV proton in this field? Neglect any slowing along its path.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 31, Problem 78 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
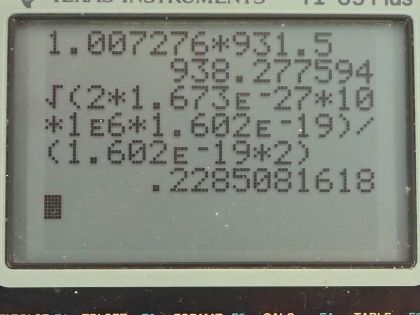
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are told that a proton moves in a 2.00 tesla magnetic field and the direction of the velocity is perpendicular to the direction of the magnetic field and we are told that the proton has an energy of 10 megaelectron volts but this begs the question what kind of energy is that? It's the kinetic energy but the reason we know is because if you convert the mass of a proton into energy, it's about 938.3 megaelectron volts of energy contained in its rest mass and that is much much greater than 10 megaelectron volts so this is to say that this number is the kinetic energy, it's not the total energy of the proton. Okay! And it's non-relativistic because this energy is so much less than the rest mass energy of the proton. So that's to say then that this kinetic energy we are given is one-half times the mass of the proton times its velocity squared and we'll need to know what this velocity is because in our formula for the force applied by the magnetic field, we have v appearing and we will need to use kinetic energy to figure out v. So the charge multiplied by its speed and then multiplied by the magnetic field strength is the magnitude of the force due to the magnetic field and there's no need for an angle here because we are told that the field and the velocity are perpendicular. This is the centripetal force that's responsible for keeping the proton traveling in its circle and so the formula for centripetal force is mass times velocity squared divided by the radius of that circle and we get to solve for r and that's the question here. So let's multiply both sides by r and divide both sides by qvB. So on the left side, we have r and on the right side, we have mv to the power of 1 divided by qB. Now v if we return to this thought about the kinetic energy if we multiply both sides by 2 over m and then take the square root of both sides we end up with this expression for the speed then: it's a square root of 2 times kinetic energy divided by mass so we substitute that in place of v here and then m times square root 2kE over m is going to be square root 2m times kinetic energy and then it gets divided by the charge times magnetic field strength. So that's the square root of 2 times the mass of a proton in kilograms times 10 megaelectron volts converted into joules by multiplying by 1 times 10 to the 6 electron volts for every megaelectron volt and then by 1.602 times 10 to the minus 19 joules per electron volt and divide that all by the charge in a proton, which is the elementary charge times 2.00 tesla magnetic field strength and the radius of curvature then is 22.9 centimeters.