OpenStax College Physics for AP® Courses, Chapter 31, Problem 59 (Problems & Exercises)

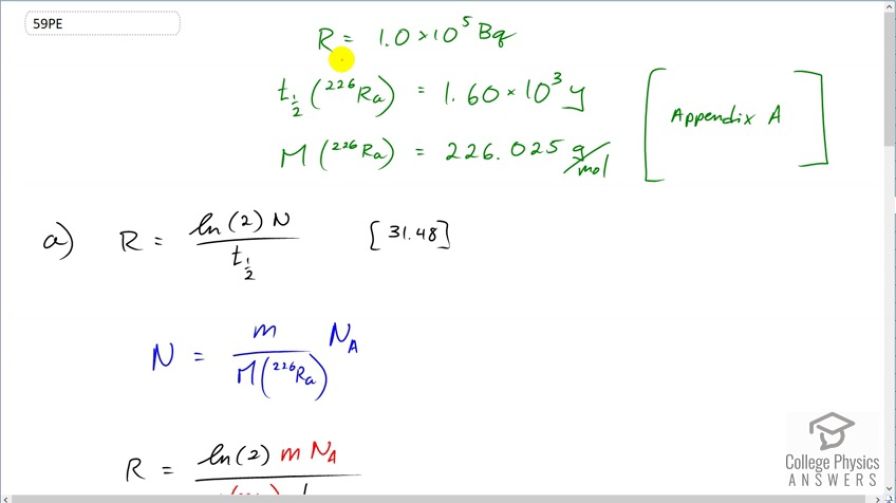
This is College Physics Answers with Shaun Dychko. We want to know the mass of radium in these World War II aircraft instrument dials. We are told that the activity, when they were first made, was 1 times 10 to the 5 becquerels. Now in order to figure out the mass that corresponds with that activity, we need to know what the half-life is of radium plus the molar mass of radium. So from appendix A, we see that the molar mass is 226.025 grams per mol and the half-life is 1.60 times 10 to the 3 years. So the activity is the logarithm of 2 times the number of atoms of radium divided by its half-life and we'll need to substitute for the number of atoms by replacing it with this because this contains the unknown mass that we want to find. So the number of atoms will be the mass divided by the molar mass times Avogadro's number and we can confirm that by writing this out as units; we can say that its grams divided by grams per mol multiplied by Avogadro's number which has units of number of atoms per mol and this works out because these grams cancel and having reciprocal mols in the denominator here is the same as having just mols in the numerator. in which case this cancels with these mols leaving us with number of atoms which is N. So N gets replaced with m times Avogadro's number divided by molar mass. So we have activity is logarithm of 2 times m N A over molar mass times half-life. Then we'll solve this for the mass little m. And so we'll multiply everything by molar mass times half-life divided by log 2 times Avogadro's number; we do that on both sides and that isolates the mass m. So the mass of radium in these instrument dials is 226.025 grams per mol—molar mass— times the half-life converted into seconds multiplied by 1 times 10 to the 5 becquerels—activity— divided by a logarithm of 2 times Avogadro's number and this works out to 2.73 times 10 to the minus 6 and it's important to know that the units will be grams because we use this molar mass expressed in grams per mol. So we are gonna convert this into kilograms because that's the mks unit so we'll multiply by 1 kilogram for every 1000 grams and we get 2.73 times 10 to the minus 9 kilograms which we can write as 2.73 nanograms because nano is the prefix representing times 10 to the minus 9. So that's a very small amount of radium. And the question in part (b) is is what is the activity of this radium now? So we know the initial activity and we are gonna multiply that by e to the negative decay constant multiplied by time; that's equation 59, and then we'll replace the decay constant using equation 37 with natural log of 2 divided by half-life and we do that here. And so the present day activity will be the initial activity of 1 times 10 to the 5 becquerels times e to the negative log 2 over the half-life—1.60 times 10 to the 3 years— and multiply that by 57 years since the instruments were made and we do not need to convert these into seconds although you could but that would be unnecessary work because these units will cancel anyway; the important thing is the units on the top and bottom are the same thing. This works out to 9.8 times 10 to the 4 becquerels is the activity now which is essentially the same as 1 times 10 to the 5 that we started with.