Question
(a) Calculate the activity R in curies of 1.00 g of .
(b) Discuss why your answer is not exactly 1.00 Ci, given that the curie was originally supposed to be exactly the activity of a gram of radium.
Final Answer
- More accurate measurements of half-life and decay rate are possible with modern instruments compared with when the curie unit was conceived.
Solution video
OpenStax College Physics for AP® Courses, Chapter 31, Problem 46 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
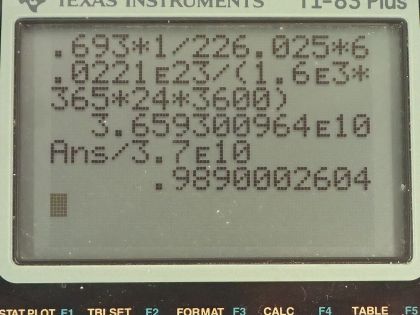
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to calculate the activity in curies of 1 gram of radium-226. We need to know the molar mass of radium-226 and it's 226.025 grams per mol, which I looked up in the appendix at the end of the textbook and the half-life of radium-226 is 1.60 times 10 to the 3 years. The activity of a radioactive substance is equal to 0.693 times the number of atoms divided by the half-life. So we need to do some work to figure out the number of atoms and that will be the mass divided by the molar mass multiplied by Avogadro's number and we'll see how the units work out in the next line here. So the activity then is 0.693 times 1.00 gram of the mass multiplied by 1 mole for every 226.025 grams this is dividing by the molar mass I am multiplying by its reciprocal so I am multiplying by the reciprocal of this number so 1 mole on the top divided by 226.025 grams in the bottom and then multiplying by Avogadro's number, which is a conversion between mols to atoms so multiplying by 6.0221 times 10 to the 23 atoms per mol. Mols cancel, grams cancel and we are left with number of atoms in this bracket, which is what we wanted for N. Then we divide by the half-life and the half-life units need to be seconds in order to have decays per second or to get units of becquerels, which we will then convert into curies. So you convert 1.60 times 10 to the 3 years into seconds by multiplying by 365 days per year and then multiply by 24 hours per day and then by 3600 seconds per hour, we end up with 3.6593 times 10 to the 10 becquerels we multiply that by 1 curie for every 3.70 times 10 to the 10 becquerels and that is 0.989 curies. Part (b) asks why is this not exactly 1 curie? Why is it not 1.00 curies since the curie unit was conceived to be the activity of 1.00 gram of radium-226? And the answer is that these days with more modern instruments, we can have more accurate measurements than compared with when the curie unit was first conceived.