Question
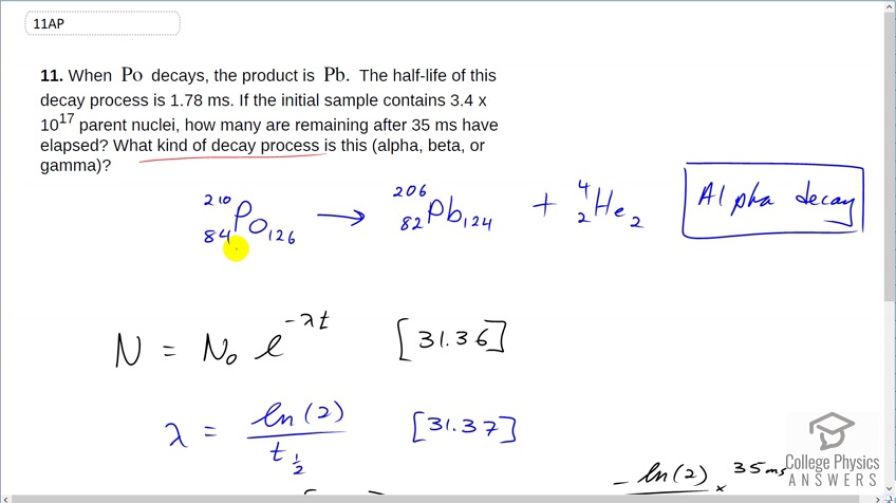
When Po decays, the product is Pb. The half-life of this decay process is 1.78 ms. If the initial sample contains parent nuclei, how many are remaining after 35 ms have elapsed? What kind of decay process is this (alpha, beta, or gamma)?
Final Answer
This is alpha decay. nuclei remain.
Solution video
OpenStax College Physics for AP® Courses, Chapter 31, Problem 11 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. Pulonium decays into lead and the first thing we'll answer is what type of decay process is this? And it's a process that involves changing an atomic number from 84 to 82; pulonium has atomic number 84 and lead has 82 protons and that process that does that is α-decay and also two neutrons are packaged along with those two protons to make a total of four nucleons bound together into this α-particle or helium nucleus. Now, the number of the parent nuclei that will remain after 35 milliseconds is given by this formula here; this is the number of parent nuclei remaining, given an initial number which in this case is 3.4 times 10 to the 17 times e to the negative decay constant times time. This decay constant is natural log of 2 over the half-life so we substitute that in and we calculate that 3.4 times 10 to the 7— initial number of parent nuclei— times e to the negative of the log of 2 divided by 1.78 milliseconds—half-life— times 35 milliseconds means there will be 4.1 times 10 to the 11 nuclei remaining after 35 milliseconds.