Question
(a) Write the complete reaction equation for electron capture by .
(b) Calculate the energy released.
Final Answer
- Please see the solution video
Solution video
OpenStax College Physics for AP® Courses, Chapter 31, Problem 42 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
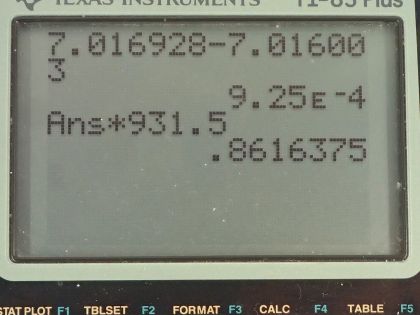
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We need to write the equation for electron capture by beryllium-7. So beryllium-7 is going to encounter an electron that will go into the nucleus and bind with a proton to turn it into a neutron— this is an unusual thing but it does happen— and we can see that the number of protons in this daughter nuclide is 1 less than the number of protons in the parent nuclide and there's 1 extra neutron compared to before but the same number of nucleons so there's still 7 nucleons in this daughter nuclide. Now because an electron was captured, this left side has an electron family number of positive 1 due to this electron and so we need to have a positive 1 electron family number on this side as well and that's accomplished with this creation of an electron-neutrino. Okay! So there we go! Part (b) asks for the energy released in this reaction so we need to take the mass difference between the products side and the reactance side and then multiply that difference by c squared— this is called the mass defect sometimes. So this mass defect is going to be the mass of the beryllium-7 atom minus the mass of the lithium-7 atom. Now you might be wondering why is there not a term for the electron mass on the left side? And the answer is that because these are atomic masses and beryllium has 4 protons and therefore 4 electrons in the atomic mass, it already has 1 additional electron included in this beryllium mass compared with the lithium's atomic mass since lithium's atomic mass includes only 3 electrons. So there are 4 electrons worth of mass in this beryllium atomic mass and only 3 electron masses included in lithium electron mass so there's already 1 additional electron mass included in the beryllium atomic mass. Okay! So we look up those masses in the appendix here: we have beryllium and then we have lithium and we copy those numbers here and we end up with 9.25 times 10 to the minus 4 atomic mass units is the difference then, we multiply that by 931.5 megaelectron volts per c squared for every atomic mass unit and then multiply by c squared in our formula and we end up with 0.862 megaelectron volts of energy released.