You can sometimes find deep red crystal vases in antique stores, called uranium glass because their color was produced by doping the glass with uranium. Look up the natural isotopes of uranium and their half-lives, and calculate the activity of such a vase assuming it has 2.00 g of uranium in it. Neglect the activity of any daughter nuclides.

Name: OpenStax College Physics Solution, Chapter 31, Problem 53 (Problems and Exercises)
Uploaded: 2019-03-08T14:02:09-08:00
Duration: 200 s
Description: You can sometimes find deep red crystal vases in antique stores, called uranium glass because their color was produced by doping the glass with uranium. Look up the natural isotopes of uranium and their half-lives, and calculate the activity of such a vase assuming it has 2.00 g of uranium in it. Neglect the activity of any daughter nuclides.

25800 Bq Note: at 0:33 in the video I say "0.27 percent", but I should have said "0.72 percent". The closed captions have the corrected text.

OpenStax College Physics for AP® Courses, Chapter 31, Problem 53 (Problems & Exercises)

In order to watch this solution you need to have a subscription.

Start free trial Log in

vote with a rating of votes with an average rating of .

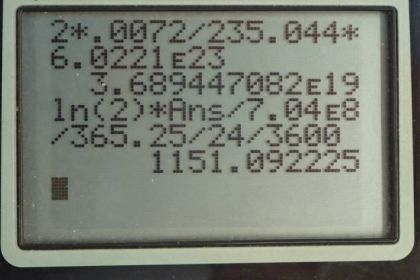
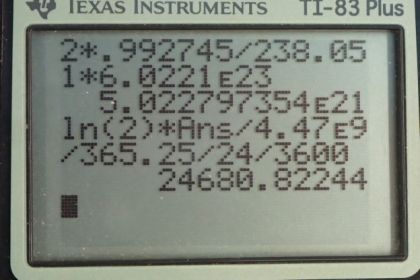
This is College Physics Answers with Shaun Dychko. In this deep red-colored crystal vase, we are told that there are 2 grams of uranium that are used to dope the glass to give it this particular color. And that is the total mass so that's the mass of the uranium-235 isotope combined with the uranium-238 isotope. And so we look in appendix A to see what the relative abundance of each of these isotopes is and we find that 99.2745 percent is uranium-238 and 0.720 percent is uranium-235 And we look up the half-life of each of those isotopes and the molar mass of each of them and we'll figure out the activity of this 2 grams by adding the activity of uranium-235 to the activity of uranium-238 and we'll figure out each of the activities knowing how many atoms there are of each which we'll calculate using the relative abundance and the molar mass. OK. So one step at a time. It's always good to, you know, write down all the information you are gonna need at the beginning so you don't have to think about this during the question because when you are doing the question, your mind is occupied by the algebra and the substitution and so on and so it's nice to have this paperwork taken care ahead of time. So activity is log 2 times the number of atoms divided by the half-life and we'll need to figure out the number of atoms of uranium-235 by taking 2 grams and then multiplying it by 0.72 percent which is as a decimal is 0.00720 and so this gives us the number of grams that are this particular isotope in this sample. Then we multiply by 1 mol for every 235.044 grams and that gives us the number of mols and then multiply by Avogadro's number to get the number of atoms. So we substitute that into our activity formula multiply by logarithm of 2 and then divide by the half-life of uranium-235 converted into seconds and this gives 1151.09 becquerels. It's important to convert the time into seconds because seconds are an mks unit—meters, kilograms, seconds— and becquerels is decays per second. OK. So then we need to figure out the activity of uranium-238 and we'll add that to the activity of uranium-235. So the number of atoms of uranium-238 is the 2 grams times the relative abundance of uranium-238 which is 0.992745 percent written as a decimal here times by 1 mol for every 238.051 grams times Avogadro's number giving us this many atoms and then that many atoms gets multiplied by log 2 and then divided by the half-life of uranium-238 converted into seconds and this gives 24680.82 becquerels. So the activity of uranium-238 gets added to the activity of uranium-235 to give a final answer of 25800 becquerels is the expected activity of the 2 grams of uranium in this vase.