OpenStax College Physics for AP® Courses, Chapter 13, Problem 70 (Problems & Exercises)

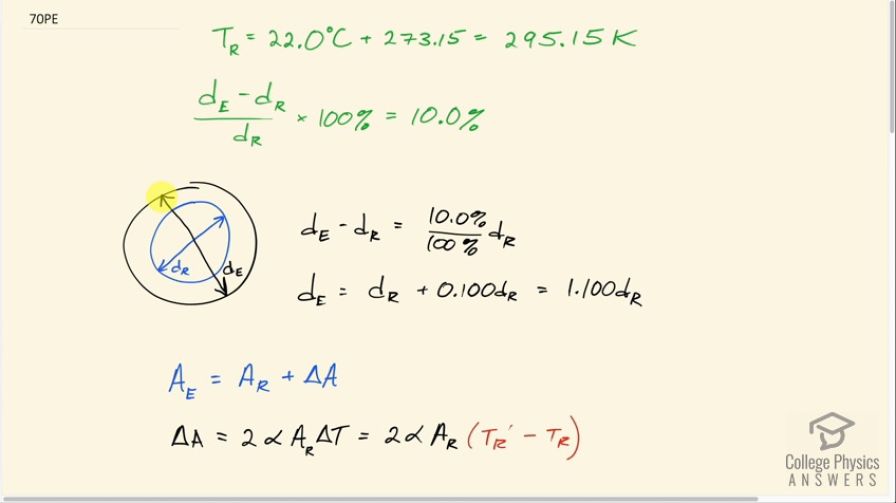
This is College Physics Answers with Shaun Dychko. An automobile mechanic claims that an aluminum rod has a diameter that is 10% less than the diameter of a hole that it's supposed to go into. So this is the diameter subscript 'e' for engine. So there's a hole in the engine with a diameter 10% more than the diameter of this rod that's supposed to go into the engine. So both the engine and the rod are aluminum and the question here is: at what temperature would this rod expand such that it would just fill the hole? So we're going to write down the things that we know. The rod we're told is initially at 22.0 degrees Celsius and have the subscript r to label it as the temperature of the rod initially. And I convert that into Kelvin by adding 273.15. And to say that the diameter of the engine is 10% more than the diameter of the rod is to say that the difference divided by the diameter of the rod times hundred percent equals 10%. So we can use this to find an expression for the diameter of the engine. And so we multiply both sides by D subscript r and divide both sides by a hundred and then we get this line here. And then we add diameter of the rod to both sides and we get diameter of the engine then is the diameter of the rod plus 0.1 times the diameter of the rod which is 1.1 times the diameter of the rod. Now that'll come in useful later. Now we have to talk about the area. So the area of the engine, the hole, is the area of the rod plus this expansion of the rod's area that's meant to occur. And so we have an expansion for the area in terms of change in temperature and it's two times the coefficient of linear expansion for aluminum multiplied by the initial rod area times the change in temperature. And the change in temperature is temperature of the rod prime minus temperature of the rod. So prime is the final temperature and this is we're going to try and find. So we can replace Delta a with this expression here and that's what I've shown in red here. And then we're also going to replace all the areas with the diameters because we know what the diameters are and the relationship between the diameters. So area of a circle in general is pi times radius squared which is pi times half the diameter squared which is pi times diameter squared over four. So we substitute for each of the areas now using this formula. So for the engine area I have pi times the diameter of the engine squared over four and that equals pi times the diameter of the rod squared over 4 plus 2 times coefficient of linear expansion for aluminum times pi times the diameter of the rod squared over four times this difference in temperatures. So the pi over 4 is a common factor, and so we're going to multiply both sides by 4 over pi to get rid of it. And then on this line the other thing that I did was I substituted for diameter of the engine and I replaced it in terms of the diameter of the rod. So the diameter the engine is 1.1 times the diameter the rod. And so that's a substitution done here. Now the diameter of the rod squared is a common factor and so we can divide both sides by D R squared and we get then that 1.1 squared equals 1 because D R squared divided by D R squared is 1, plus 2 times alpha times T R prime minus T R. Then we subtract 1 from both sides and then divide both sides by 2 times alpha and we get this line here after switching the sides around. T R prime minus T R equals 1 point 1 squared minus 1 all over 2 alpha. And then add T R to both sides and we'll get an expression that will help us find the answer. So T R prime is the initial temperature of the rod plus 1 point 1 squared minus 1 over 2 alpha. So that's 295.15 Kelvin, the initial temperature of the rod, plus one point one squared minus one over two times this coefficient of linear expansion for aluminum that we found in table of 13.2. And it's 25 times10 to minus 6 per Celsius degree. And this works out to 4.50 times 10 to the 3 Kelvin. Now the boiling temperature of aluminum is 2.74 times 10 to the 3 Kelvin. So this exceeds the boiling temperature of aluminum. So this is clearly an unreasonable temperature the rod and engine would turn into gas at this temperature. So it's unreasonable to presume that the rod could expand its diameter by 10%; that's a very large expansion. It would expand some number much less than this.