Question
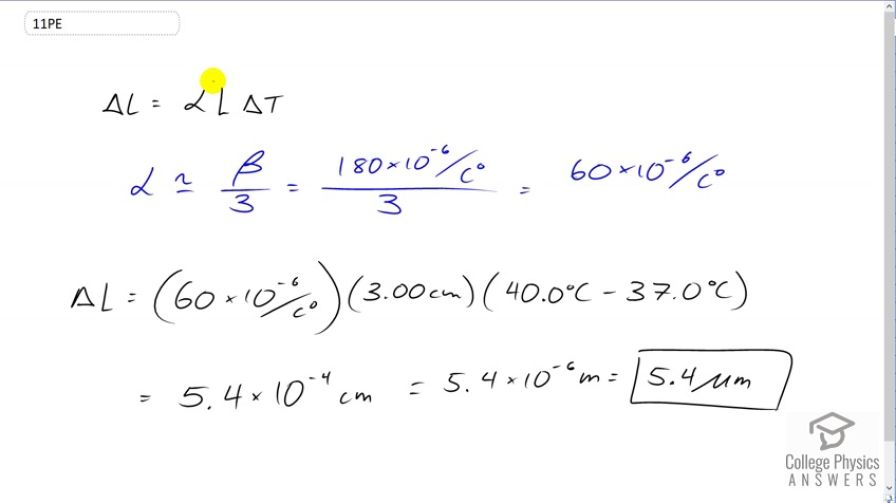
What is the change in length of a 3.00-cm-long column of mercury if its temperature changes from to , assuming the mercury is unconstrained?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 13, Problem 11 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The change in length of the Mercury column will be its linear coefficient of expansion times its original length times the change in temperature. Now, we're not actually given a linear coefficient of expansion because Mercury is a liquid. And so normally, you think of its volume expanding. But in this case, basically it's confine to a container of some kind and it's going to be expanding linearly. And we can calculate an alpha based on the volume coefficient of expansion by dividing it by three. And the way I remember it is that this volume coefficient of expansion involves three different dimensions x, y and z. And so dividing it by three gives us the coefficient of expansion in one dimension. And so we have 180 times ten to the minus six per Celsius degree volume coefficient of expansion for Mercury divide that by three and we get 60 times ten to the minus six. So the change in length of the Mercury column will be 60 times ten to the minus six per Celsius degree times the original three centimeters times the change in temperature of forty degrees Celsius final temperature minus 37 degrees Celsius initial temperature. And this gives 5.4 times ten to the minus four and the units are centimeters because I didn't bother converting that into meters. While the prefix centi means times ten to the minus two. And so ten to the minus two times ten to the minus four makes ten to the minus six. And this is the prefix which is micro. So this is going to change in length by 5.4 micrometers.
