Question
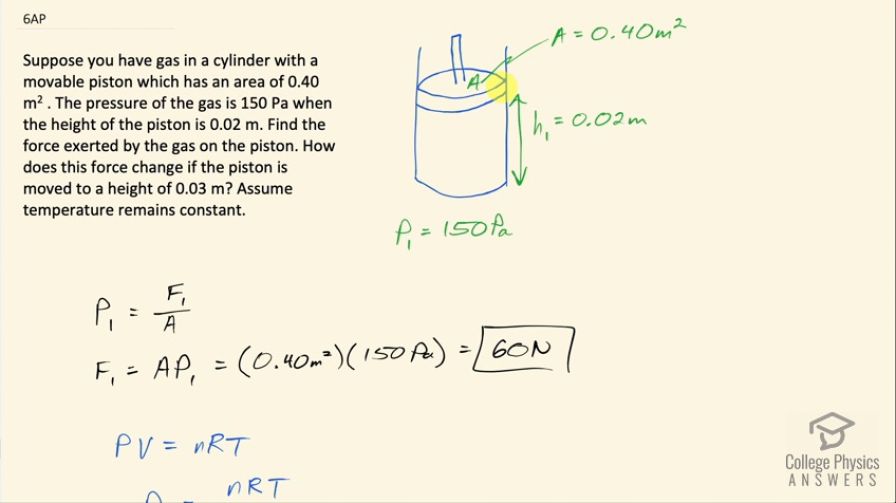
Suppose you have gas in a cylinder with a movable piston which has an area of . The pressure of the gas is 150 Pa when the height of the piston is 0.02 m. Find the force exerted by the gas on the piston. How does this force change if the piston is moved to a height of 0.03 m? Assume temperature remains constant.
Final Answer
At , .
At , .
Note: While the answers are correct, at 0:39 Shaun mistakenly said when it should be , and at 2:21 it should be over . The closed captions have been corrected, and the final answers are nevertheless correct.
Solution video
OpenStax College Physics for AP® Courses, Chapter 13, Problem 6 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a cylinder with a piston that's movable that has some area of 0.40 square meters and the piston is initially at a height of 0.02 meters—we'll call that height one— and that height one, it has a pressure one of 150 pascals and the first question is what is the force on this piston? So pressure is force divided by area and we can solve for force by multiplying both sides by area and the force in this first scenario then is the area multiplied by the first pressure so that's 0.40 square meters times 150 pascals, which is 60 newtons. And then the piston will be moved to a new height of 0.03 meters— we'll call that h 2— so h 2 is 0.03 meters, what will the force on the piston be then? We need to find out what the pressure will be in that second scenario. We use the ideal gas law and we use the assumption that temperature is constant so this T does not need a subscript. So we have pressure one is the number of moles of gas times this gas constant times T divided by this first volume and the pressure in the second case is the same number of moles and same temperature divided by this new volume V 2 and we can take the ratio of these pressures and that's going to be nRT over V 2 multiplied by the reciprocal of P 1 so V 1 over nRT and the nRT's cancel leaving us with V 1 over V 2 and the volume for its cylinder is its height multiplied by the area of its end and the area does not need a subscript because it's the same piston in both cases and so this is a factor that cancels and we are left with the ratio of pressures equals the ratio of the height of the piston but the other way around so P 2 over P 1 is h 1 over h 2 and we can solve for P 2 by multiplying both sides by P 1 and we have pressure two then is pressure one times height one divided by height two. So when we find the force in the second case, it's gonna be the area times pressure number two based on this definition of pressure and we substitute for P 2 as P 1 times h 1 over h 2. So that's 0.40 meters squared times 150 pascals times 0.02 meters divided by the second height of 0.03 meters and that will be 40 newtons.
