Question
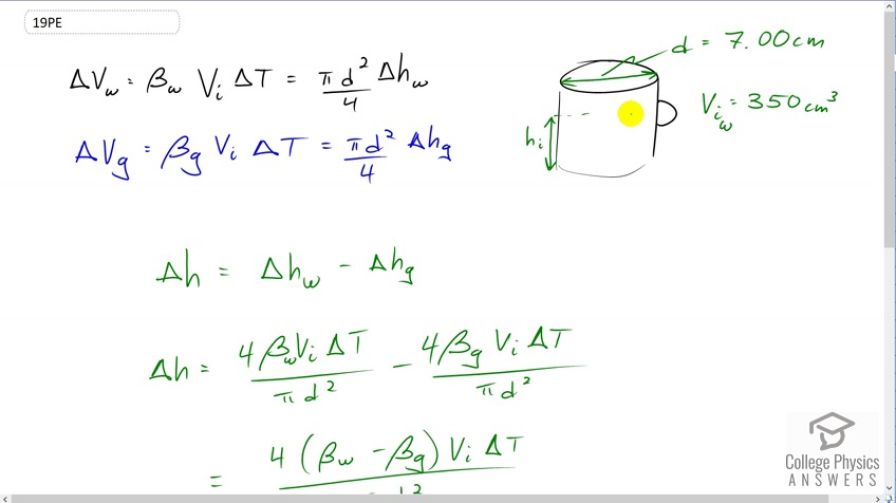
A physicist makes a cup of instant coffee and notices that, as the coffee cools, its level drops 3.00 mm in the glass cup. Show that this decrease cannot be due to thermal contraction by calculating the decrease in level if the of coffee is in a 7.00-cm-diameter cup and decreases in temperature from to (Most of the drop in level is actually due to escaping bubbles of air.)
Final Answer
Only of the drop is accounted for by thermal contraction, leaving resulting from air bubbles leaving the coffee.
Solution video
OpenStax College Physics for AP® Courses, Chapter 13, Problem 19 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This coffee cup is filled to some initial volume here and some initial height with some hot coffee and the hot coffee in the cup will reach some equilibrium temperature. You know the heat capacity of water is quite high o it can probably heat up this coffee cup without actually reducing its temperature very much at all. So we'll assume that both the coffee cup and the coffee both begin at 95 degrees Celsius. Now as the two things cool, that is the glass in the coffee cup and the water which is basically what coffee is -- as these things cool they will have thermal contraction at different rates. So they will both thermally contract different amounts and since the coefficient of expansion of volume expansion for water is greater than that of glass, the water will contract more. That's going to be responsible for a bit of the change in the water level or the coffee level in the cup. So we'll figure out how much of the -- the total change is three milliliters or sorry, three millimeters I should say, and we're going to see how much is thermal contraction contributing to that drop. Okay. So the change in volume of the coffee which I am going to refer to as water from now on, because we're using all this -- we're using the coefficient of volume expansion for water here. The change in volume of the water is this coefficient times the initial volume times change in temperature, which also is going to be the area of the circle at the end of this cylindrical cup, pi times diameter squared divided by four, multiplied by the change in height of the water. We're going to assume that the diameter of the cup basically stays the same as the temperature changes. The diameter is the same for the water as it is for the glass and so it does not need a subscript. So the change in volume of the glass is the same idea, only we're using the volume coefficient of expansion for glass instead of for water. Now, so you can see there's a change in height for each of these things, change in height of the water and change in height of the glass, and the change in height or the change in level of the coffee in the cup is the difference in the changes in height of the water and the glass. So let's solve this for delta h w here and we'll multiply both sides by four over pi times diameter squared. We'll multiply this also by four over pi times diameter squared and we get delta h w in that case, and that's what I've written here. So this is four beta w v i delta t over pi d squared. Then likewise for glass we have that written here for delta h g, the change in height of the glass as it cools. There are a lot of common factors here and you can factor them out. We have four times the difference in the volume coefficients of expansion, then times by the initial volume times delta t over pi d squared. Not sure if I mentioned that these two volumes will start out as the same. Now of course the volume of the cup will be greater than the volume of the coffee to begin with because normally one leaves a space of air at the top of the cup to avoid spilling when you carry it. But that's not important here. We're considering just the portion of the cup which is in contact with the coffee. So the volume of glass that we're concerned with initially, is the same as the volume of the coffee. Okay. So, we have four times the difference in the volume coefficients of expansion for water and glass, and then times by the initial volume of 350 cubic centimeters and I did not change this into meters because we're going to be dividing by centimeters squared. So long as we're consistent with units we can do what we want. There is no other factors in this equation that require us to use meters. These things are in units of per Celsius degrees and that's going to get multiplied by the Celsius degrees here causing those to cancel. We're left with centimeters cubed divided by centimeters squared which will give us an answer in centimeters to the power of one. So we multiply by the change in temperature of 95 degrees Celsius initial temperature, minus 45 degrees Celsius final temperature, and divide by pi times the diameter of the cup in centimeters squared. This gives 0.832 millimeters. The three millimeter drop in the level of the coffee is not accounted for completely by this thermal contraction. There is still 2.17 millimeters unaccounted for. The question tells us that this is a result of air bubbles leaving the coffee.
