OpenStax College Physics, Chapter 12, Problem 20 (Problems & Exercises)

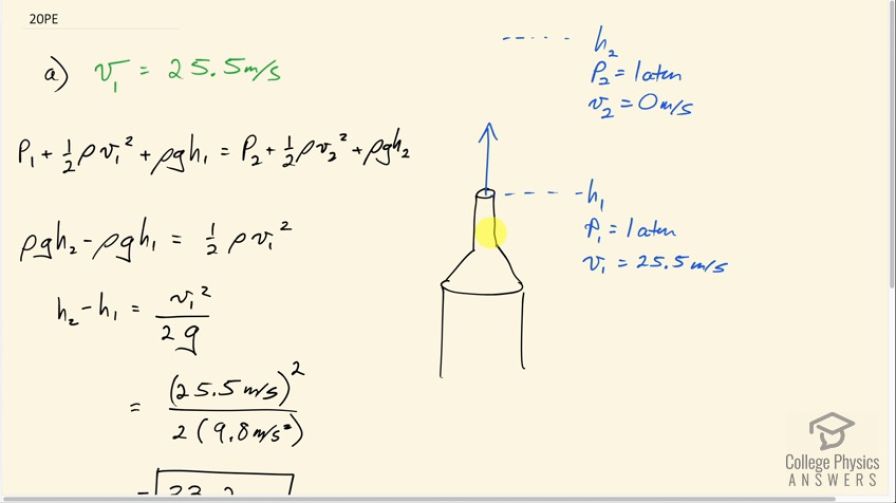
This is College Physics Answers with Shaun Dychko. In example 12.2, we found that the water squirts out of this hose when it has a narrow nozzle on it at a speed of 25.5 meters per second. So this is the initial position here where the water emerges from the nozzle and it has height h one, we'll call it. And it has a pressure there, which is one atmosphere because it's now out of the nozzle. This is just when it's out of the nozzle, until it's in the atmosphere. So it has to have atmospheric pressure and it has the speed that we're given from the example. And then the water goes up to this maximum height up to here. We call that height h two and it will have a pressure P two, which is still one atmosphere. And its speed at that point will be zero meters per second when it gets to the maximum height. And labelling it this way corresponds to all the terms in the Bernoulli’s equation. And so we can say that P one and P two are the same. And so we can subtract them from both sides and V two is zero. So this term disappears and we can subtract rho g h one from both sides. And then and then we switch the sides around and we get rho g h two minus rho g h one equals one half rho V one squared. Now, the density of air and the acceleration of gravity can be factored out. And then we can divide both sides by it. So divide both sides by rho and g and the rho cancel everywhere. And we're left with h two minus h one is V one squared over two times acceleration due to gravity. So this difference in height is the height that the water goes from the nozzle to the top. So that's 25 and a half meters per second squared divided by two times 9.8 meters per second squared, which is 33.2 meters. And then in part B, we have a different speed. When there's no narrow nozzle on it and it emerges at 1.96 meters per seconds and we square that divided by two times 9.8 meters per second squared gives a height of about 19.6 centimeters or 0.196 meters.