OpenStax College Physics, Chapter 12, Problem 18 (Problems & Exercises)

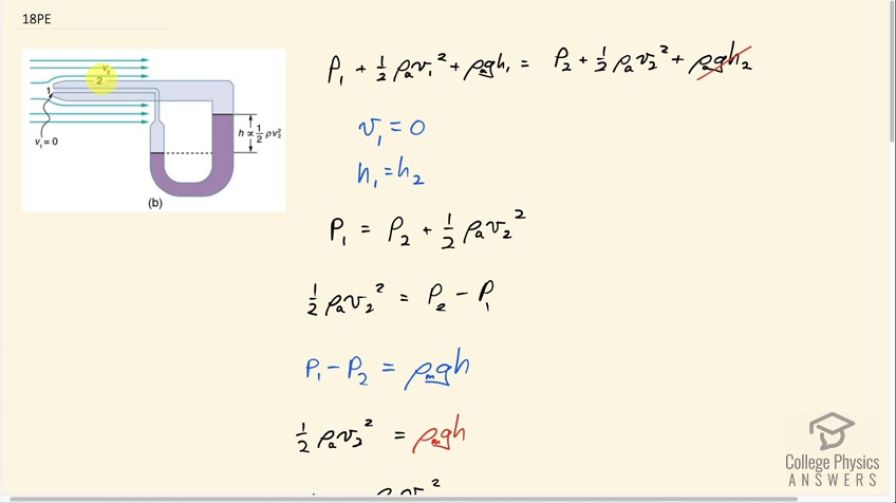
This is College Physics Answers with Shaun Dychko. This pitot tube is going to measure the speed of this wind travelling past this whole apposition to the top of this tube. And that wind speed, we’ll call V two and that's going to result in a low pressure in this section of air here and because this wind is moving and fast moving fluid has lower pressure. That's the Venturi effect. And you can see that moving air sucks is a way of remembering as venturi effect and the wind when its at this position… at position one is going to stop because it's just hitting a dead end there. This tube is closed and and it's going to impact this fluid in this manometer. And this higher pressure in this section of tubing will cause this fluid to go down here. And it's also being sucked up here in this low pressure section here, resulting in this height h which is indicative of the pressure difference between these two sections of tubing. And the question here is asking, by what factor does this wind speed need to increase such that the height of this manometer fluid doubles? So we start with the Bernoulli's equation, which says that the pressure at position one plus one half times the density of the air times its speed at position one squared, plus the density of air times g times the vertical position at the vertical height at position one, h one. All of that equals the corresponding things at some position two. Now, h one and h two are essentially the same. The height difference between this hole and this opening here is minimal, negligible. So these terms are the same and it can be subtracted from both sides and the speed at position one is zero. So this term disappears and we're left with this expression. So the pressure at position one equals the pressure at position two plus one half times the air density times its windspeed squared. And we can solve for this term and say that it's equal to P two minus P one. This P two minus P one change in the numbers there, the order doesn't really matter. It's the magnitude of that pressure difference is the density of this manometer fluid and which is why I have the subscript m therefore the density of the manometer fluid times g times this height. And that can be substituted in place of P two minus P one, which I've done here. And this gives an expression for height of the manometer fluid in terms of the windspeed and in terms of these other things to do with the density of air, density manometer fluid and g. So, the height in general is this, and we can consider the height and scenario A with some wind speed A as density of air times, the speed A squared over two times the density of the manometer fluid times g and then at some wind speed, B, it'll be the same expression, but with a V b instead of a V a and height B we're told is two times height A. And so we substitute that in place of h b and write two h a and then h a is this expression. So we make that replacement here and we have the most or a lot of factors are the same. So we can divide both sides by density of air multiply both sides by two, multiply both sides by density of the manometer fluid and g. And we're left with V b squared switching the sides around, we have V b squared equals two V a squared. In which case, we take square root of both sides. We can say that V b is square root two times V a. So that means the windspeed we need to increase by a factor of square root two to double the height in the manometer. And we notice that does not depend on what the densities of the moving fluid or air is, nor the density of the manometer fluid.