OpenStax College Physics, Chapter 12, Problem 44 (Problems & Exercises)

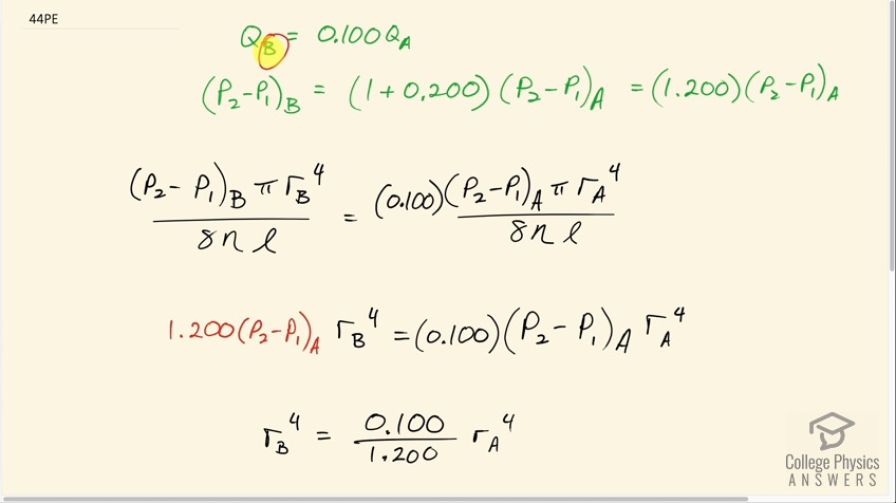
This is College Physics Answers with Shaun Dychko. We're told that an artery is blocked by a blood clot causing the volume flow rate after the blood clot, which we label scenario ‘B’ to be 10% of the volume flow rate before the blood clot Q A and 10% is being written as a decimal here is 0.100. So we're also told that the pressure difference has increased by 20%. So we add this 20% as a decimal to the original 100%, which is one. And that makes the pressure difference in scenario ‘B’, 1.2 times the pressure difference in scenario ‘A’. So we're going to write the Perseus law for the volume flow rate in terms of this pressure difference and radius of the tube and a bunch of other things. And so we have pressure, difference scenario ‘B’ times pi times the radius of the artery to the power of four divided by eight times the viscosity of blood times the length of this blood vessel. And that's all scenario ‘B’ after the blood clot. And that's going to equal 10%, which is 0.1 times Q A. That's what all this is. The pressure difference in scenario ‘A’ times pi times the radius of the artery in the scenario ‘A’ to the power of four divided by eight times new times l. This viscosity and this length do not get subscripts because of the same in both scenarios. And they become factors that cancel. So we can simplify things by multiplying both sides by eight new l over pi. And then we get this line here where I've also made a substitution for pressure difference in scenario ‘B’ by replacing it with 1.2 times pressure difference in scenario ‘A’. So we have all of this times r B to the power of four equals 0.1 times the pressure difference in scenario ‘A’ times r A to the power of four. This pressure difference is now something that can cancel on both sides. So we divide both sides by that. And then we also divide both sides by 1.2 in order to isolate r B to the power of four. And we’re left with this line here, r B to the power of four is 0.100 divided by 1.2 times r A to the power of four. And then we raise both sides to the exponent one quarter in order to get r B. So, r B is going to be 0.100 over 1.2 to the power of one quarter times r A. And that's 0.537. And so the radius has been reduced by a factor of 53.7%, which should be somewhat shocking because the radius is only is half of what it used to be, but the flow rate is one tenth of what it used to be. So this flow rate is very sensitive to changes in radius. And so a relatively small change in radius, multiplied by a factor of a half, basically causes a huge change in volume flow rate to reducing it by 90 percent. So and that's because of this dependence to the power of four on the tube radius.