Question
What is the greatest average speed of blood flow at in an artery of radius 2.00 mm if the flow is to remain laminar? What is the corresponding flow rate? Take the density of blood to be .
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 57 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
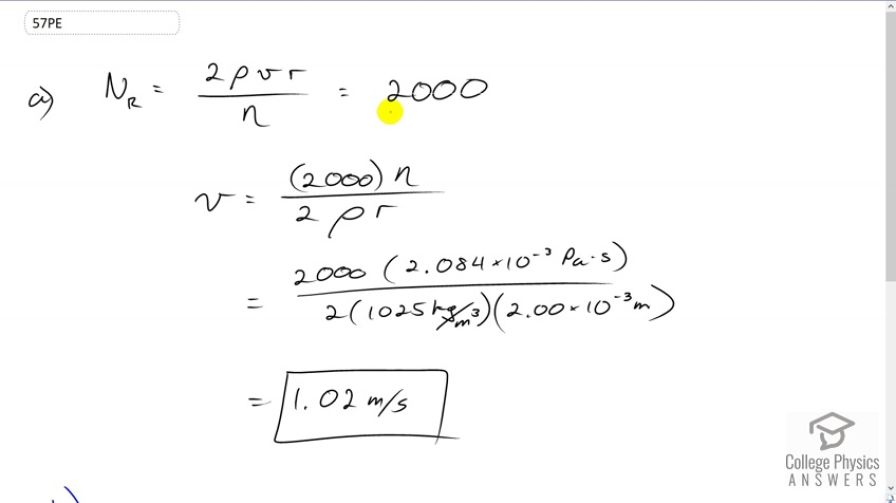
This is College Physics Answers with Shaun Dychko. We’ll find the greatest average speed for non-turbulent blood flow by using this Reynolds number formula and it has to equal at the most 2000 for laminar flow. And so we rearrange this to solve for v by multiplying both sides by nu over 2 rho r, and we get this speed then is this 2000 Reynolds number threshold of turbulent or laminar flow, times the viscosity of the blood, which we have to use the value from the data table given to us for 37 degrees Celsius, divided by two times the density of whole blood times the radius of the blood vessel. So we have 2000 times 2.084 times ten to the minus three Pascal seconds, divided by two times 1025 kilograms per cubic meter times two times ten to the minus three meters, and we get 1.02 meters per second. Now the volume flow rate is going to be the cross sectional area of the blood vessel times that speed, so that’s pi times radius squared times speed. That’s pi times two millimeters squared, converted into meters of course, multiplied by this speed which I’ve written with a whole bunch of extra digits to avoid intermediate rounding error, and we get 1.28 times ten to the minus five cubic meters per second.
