Question
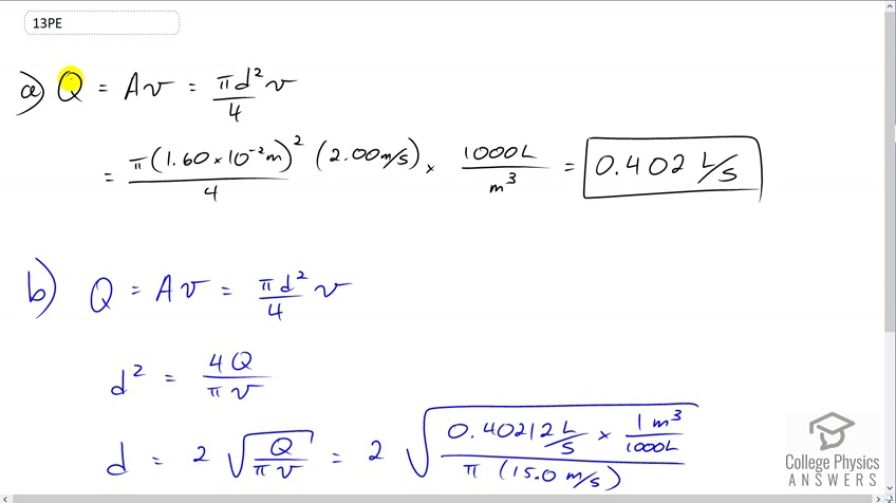
Water is moving at a velocity of 2.00 m/s through a hose with an internal diameter of 1.60 cm. (a) What is the flow rate in liters per second? (b) The fluid velocity in this hose's nozzle is 15.0 m/s. What is the nozzle's inside diameter?
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're given the speed of a fluid and the internal diameter of the hose. And so we can use both of those pieces of information to figure out the volume flow rate. Now, the area is going to be Pi times the diameter of the hose squared over four and we'll multiply that by the speed. And taking care of a little bit of unit conversion, as well. So we have 1.6 times ten to the minus two meters. Convert this 1.6 centimeters into meters and square that diameter times by Pi divided by four times by 2.0 meters per second and then change that into Liters, as well, because we want to have Liters per second in our answer, we're told. So we have multiplied by 1000 liters for every cubic meter and we end up with 0.402 Liters per second. Now, in the hose's nozzle, the speed is different. That's not two meters per second anymore. It is 15 meters per second. And so the question is what must the diameter of the nozzle be? We'll the flow rate has to be the same as it was before because for an incompressible fluid like water, the flow rate has to be the same at all points along the hose regardless of how its diameter changes. And so we say Q is area times speed and then Pi d squared over four again substituted for area. And then we'll solve this for d now. And so we multiply both sides by four over Pi times speed. And so we have d squared is four Q over Pi V and then we'll take the square root of both sides. And then we get d as two times square root Q over Pi V. So that's two times square root of 0.40212 Liters per second converted into cubic meters per second because we want to have consistent units on the top and bottom here. And then bottom has units of meters per seconds, so we need to have cubic meters on the top. So we have 0.40212 Liters per second times one cubic meter for every meter divided by Pi times 15 meters per second. And this gives 0.00584 meters which is 5.84 millimeters.
