Question
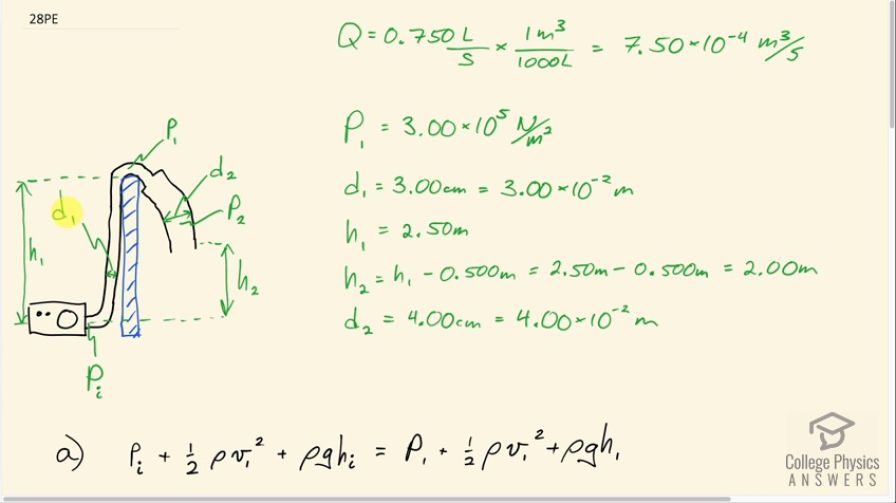
A sump pump (used to drain water from the basement of houses built below the water table) is draining a flooded basement at the rate of 0.750 L/s, with an output pressure of
. (a) The water enters a hose with a 3.00-cm inside diameter and rises 2.50 m above the pump. What is its pressure at this point? (b) The hose goes over the foundation wall, losing 0.500 m in height, and widens to 4.00 cm in diameter. What is the pressure now? You may neglect frictional losses in both parts of the problem.
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 28 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A sump pump is pumping some water from this basement up through this pipe with a diameter of d one to the top of the foundation wall and there's gonna be a pressure P one at this position and then the water continues through this pipe. But the pipe expands into a larger pipe with diameter d two and then it will have a new pressure P two here that we also have to find. So we're gonna find pressure P one and P two. The height of P one is this height h one above the pump. And that is two and a half meters and then we're told that the pipe goes half a meter down after it crosses over the foundation. And so this h two this height compared to the pump is gonna be the height one minus that half meter down from the top of the foundation. So it's two and a half minus 0.5 which is two meters. This is two meters above the pump level and the the diameter in the second portion of pipe here is four centimeters. And we convert that into 4.00 times ten to the minus two meters and doing all of our unit conversions in the step of writing down the things that we know in order to avoid thinking about this unit conversion business when we're thinking about other stuff. So our volume flow rate through the pipe is the same and every portion of pipe that's what the equation of continuity says since liquids are incompressible, you have to have the same quantity or same volume flowing through each portion of this pipe in the same time. And that's 0.75 litres per second and we'll convert that into cubic meters per second by multiplying by one cubic meter for every 1000 liters and that 7.50 times ten to the minus four cubic meters per second. The pressure initially, I call this P i for initial at the pump is 3.00 times ten to the five pascals or newtons per square meter whichever way you like to write those units. And so there we go. That's what we know. And so the the wording in the question is that the pump has an output pressure of three times ten to the five pascals and that's what we take to be the pressure here. Good. Now we're ready. So first part in A is to figure out the pressure at the top of the foundation. So, Bernoulli’s equation tells it that the initial pressure here plus one half times the density of the water going through this pipe multiplied by its speed to the pipe squared plus the density of water times acceleration due to gravity times the initial height equals the same three corresponding terms but at the top of the foundation wall. So it's going to be P one which we're going to find plus the same term because since the diameter of this pipe is the same from the pump all the way to the top of the foundation wall. The speed of the fluid will be the same. So these terms are the same and they'll end up subtract them from both sides and so they will cancel each other out. So it just makes zero. And then this will be different though because there's a new height h one here. So P one is going to be this initial pressure at the pump plus this term minus this term here because we're going to subtract it from both sides and then that isolates P one and then we'll switch the sides around and we get the P one is P initial plus rho g h i minus rho g h one and rho and g pair of common factor will be factored out and so we have three times ten to the five newtons per square meter initial pressure at the pump level plus 1000 kilograms per cubic meter density of water times 9.8 newtons per kilogram gravitational field strength times initial height which we can choose to be zero in which case h one becomes two and a half compared to each initial and so we have zero minus two and a half meters and this is gonna make this term negative which will reduce this number here to a smaller pressure of 2.76 times ten of the five pascals and so we expected the pressure up here to be smaller than the pressure at the pump because the pressure of the pump also includes the pressure caused by this vertical column of fluid here and P one does not include that vertical column above it… And then part B asked us to find the pressure here in this larger diameter portion of pipe that is 0.5 meters below the top of the foundation or in other words two meters above the pump level. So, using Bernoulli’s equation again but this time this dynamic term due to the pressure due to the fluid moving is not the same on both sides. Because since the diameter of piping changes the speed of the fluid will change. So that makes this question a bit more complicated. So it's Bernoulli’s equations just the same though it's P two plus one half rho V two squared plus rho g h two and the subscript two is referring to things at this position so we're talking about the speed of the fluid V two here, we're talking about the pressure P two there and we're talking about the height h two there and then that equals the same three terms any other place. Let's to the pump level to be the place we're comparing to. So we can use the initial pressure at the pump plus one half rho the speed of the water through that first portion of pipe squared plus rho g h i and h i we know is zero. So this term will become zero. Now we need to make substitutions for these speeds because we don't know the speeds but we do know the volume flow rate. We're given that. And so the volume flow rate is the cross-sectional area of the tube times the speed. And so we can divide both sides by A to solve for V. And so the speed in Section 1, the first part of the pipe is gonna be Q which I do not put a subscript on because the volume flow rate is the same in all parts of the pipe but it divided by A subscript one though since this is a cross-sectional area the first person to pipe and this cross-sectional area is pi times radius squared and radius is half the diameter and we're given diameter. So I put in d one divided by two and this works out to four Q over pi d one squared. V two by the same logic is gonna be four Q over pi times d two squared. So, we can substitute for V one and V two in Bernoulli’s equation and we do that here showing the substitutions in red so we have four Q over pi d two squared is a substitution for V two and that is in turn squared and same with V one is substituted. And so we have P two plus eight rho Q squared and that's an eight because four square is sixteen but it's divided by these twos so that makes eight rho Q squared over pi squared d two to the power of four, plus rho g h two equals P initial plus eight rho Q squared over pi squared d one to the power of four plus rho g h i. So, we're gonna solve for P two by subtracting these two terms from both sides and I'm also going to factor some things out at the same time so I'm subtracting eight rho Q squared over pi squared d two to power of four from both sides and this eight rho Q squared over pi squared is a common factor between these two terms so we can factor it out here and we end up with one over d one to the power of four minus one over d two to the power of four and then add to that rho times d as a common factor times h i minus h two because this rho g h two is being subtracted from both sides and we will left with this line here. So, then we can plug in numbers and get our answers. So, we have three times ten to the five newtons per square meter output pressure of the pump plus eight times the density of water times the volume flow rate of 7.5 times ten to the minus four cubic meters per second squared divided by pi squared times one over the diameter in the first portion of pipe in meters so that’s three times ten to the minus two meters to the power four minus one over four times ten to the minus two meters to the power four, that's the diameter in the second portion of pipe plus 1000 kilograms per cubic meter times 9.80 newtons per kilogram times the pumps height of zero minus the position two height of 2.00 meters. This works out to 2.81 times ten to the five Pascal's would be the pressure in the second portion of the tubing.

