Question
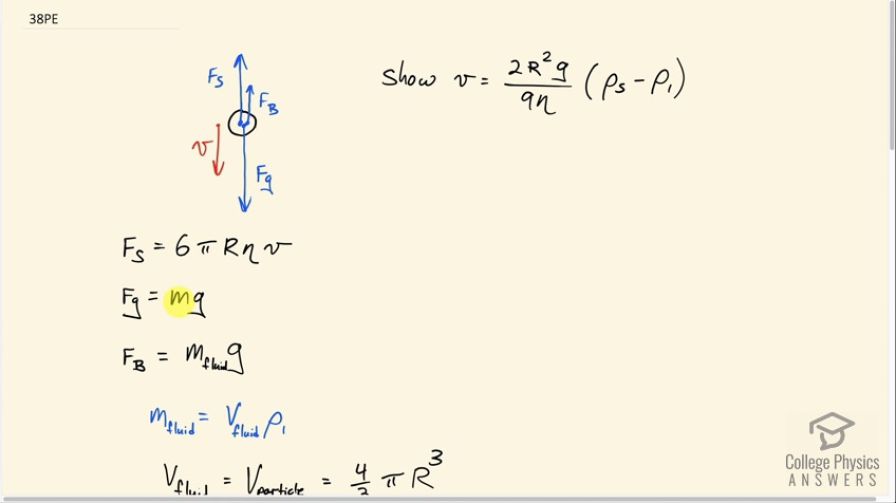
A spherical particle falling at a terminal speed in a liquid must have the gravitational force balanced by the drag force and the buoyant force. The buoyant force is equal to the weight of the displaced fluid, while the drag force is assumed to be given by Stokes Law, . Show that the terminal speed is given by , where is the radius of the sphere, is its density, and is the density of the fluid and the coefficient of viscosity.
Final Answer
Please see the solution video.
Note: Where I've said the letter "nu" for the viscosity , I should have said "eta".
Solution video
OpenStax College Physics, Chapter 12, Problem 38 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. A spherical droplet is falling in a fluid and gravity is directed downwards and it has a formula, mass of the droplet times g and upwards is going to be two forces. One is the buoyant force this buoyant force will equal the weight of the fluid displaced or its mass of the fluid displaced times g and then there will also be this drag force which is given by Stokes Theorem. And this drag force will be equal to six times pi times the radius of the droplet times the viscosity of the fluid that is flowing through times its velocity. And this will be a terminal velocity when the drag force plus the buoyant force and total equals the gravity force downwards. Because in that case, there will be no more acceleration down. If these forces balance out, up and down here, in other words, if there's zero net force. So we're gonna show that this terminal velocity is two times the radius of the droplet squared times acceleration due to gravity divided by nine times the viscosity of the fluid that is falling through, which could be air or or could be water, whatever it is at times by the density of the material that the sphere is made out of, minus the density of the fluid. So we've had written down these expressions for each of the forces and we're going to make substitutions using the radius of the sphere and these densities. So the mass of the fluid displayed, we're gonna work on this buoyant force formula first. The mass of fluid displaced is the volume of the fluid times the density of the fluid and using a subscript ‘one’ there because that matches what's shown in this formula that we're given. And the volume of the fluid displayed is going to equal the volume of the particle because the particles completely submerged. And so that's when this becomes true, is when the particle submerged. And that's going to be four thirds pi times the particle’s radius to power three. And then we can substitute that volume in here for the mass of the fluid. And then the buoyant force becomes four third pi times radius of this sphere cubed time density of the fluid times g. And so that's all the work we need to do on the buoyant force, because now we've expressed it in terms of the radius of the sphere and the density of the fluid. The Stokes force… Well, I'm just repeating it from up there. Nothing new there. So we can replace each of these by saying, you know, the Stokes drag force upwards plus the buoyant force upwards equals the force of gravity down. So that's six pi R new V plus four third pi R cubed density of fluid times g equals the mass of the particle times g. Now, this mass of this particle needs to have some work done on and it's going to be the volume of the particle times the particles density, density of sphere. That's why there's an ‘S’ there for sphere and the volume is four thirds pi R cubed, which I usually draw in red to show that's a substitution. Here we go. And then this can be written in place of m. Now I'm doing two things here. On the one hand I'm replacing m with all of this. And that's what I've done here. And then I'm also subtracting this four thirds pi R cubed rho one g from both sides. And the reason we're doing that is we want to isolate V on one side. And so we have V times a whole bunch of factors. At this point. So we have six pi R new V equals four third pi R cubed rho S g minus four third pi R cubed rho one i>g and then this four third pi R cubed g is a common factor between this term and this term. So we factor it out. And then write that multiplied by density of the sphere minus density of the fluid. Then we divide both sides by six pi R new to isolate V. And the four and the six reduced to two thirds and then three times three is nine. And then the pi cancel, i>R cubed divided by R is R squared and the g is there. And then we have this factor new in the bottom as well and times by rho S minus rho one. And there we go. That's what we want to demonstrate that the terminal velocity for a spherical particle falling in a fluid is two times the particle radius squared times g divided by nine times the density of the fluid times the difference between this sphere density and the fluid density. And we can say quod erat demonstrandum, quite easily done.