OpenStax College Physics, Chapter 12, Problem 34 (Problems & Exercises)

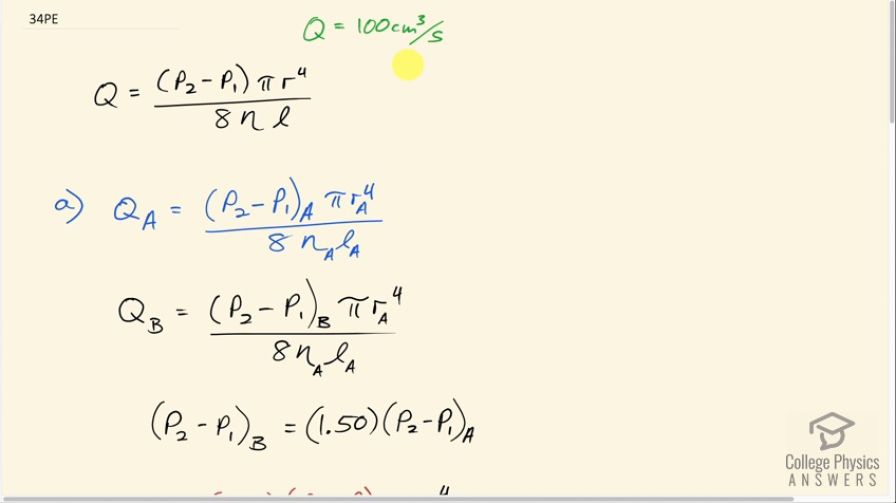
This is College Physics Answers with Shaun Dychko. We're told that fluid flows through a tube at a rate of 100 cubic centimeters per second. And then in parts, A, B, C, D and E; we’re given changes to the tube and asked to find out what would the new flow rate be given those changes. So the formula for volume flow rate is the pressure difference between the ends of the tube times pi times the radius of the tube to the power four divided by eight times the viscosity of the fluid flowing through the tube multiplied by the tubes length here. So in case A the original 100 cubic centimeters per second scenario we have, the flow rate is P two minus P one. And we call that subscript ‘A’ for the pressure difference in scenario A times pi times the radius in scenario A to the power four divided by eight times the viscosity ‘A’ times length ‘A’, And then after the changes made we'll have a new flow rate which we'll call Q subscript ‘B’ and we'll replace whatever we're meant to in that part with subscript ‘B’ here. So the pressure difference in part A, we're told is increased by a factor of 1.5. So the pressure difference in scenario B is 1.5 times the pressure difference in scenario A. So we can replace the pressure difference with 1.5 times P two minus P one, ‘A’. And this whole thing here is Q A. Until we see that we have 1.5 is multiplied by Q A, so that tells us how to find Q B, 1.5 times 100 centimeters cube per second, which is 150 centimeters cubed per second. Okay, so in part B we're going to do it just in one step. Now, instead of writing a subscript ‘B’ here and then saying, well, this is some multiple of that and then substitute it in, we are just going to do the substitution right away. So the new flow rate here is going to be the same as before. But we're replacing the viscosity of fluid with three times original viscosity ‘A’. And so this ends up being Q A because that's what all of this is and these are everything except for the number three there in the denominator. All of that makes Q A and it's being divided by three. So our answer now is then 100 cubic centimeters per second divided by three, which is 33.3 cubic centimeters per second. In part C, the tube is replaced by one having four times the length. So we have our expression for Q A but we're replacing the length with four times l A and so that gives us volume flow rate ‘B’ with a substitution of four l A here. And this is Q A divided by four then and that's going to be 25 cubic centimeters per second. In Section D, our change is to have a tube with a radius 0.1 times the original. So I'm replacing r A with 0.1 times r A and this factor is being raised to the power of four. And we have 0.1 to the power four multiplied by Q A since all of this other stuff together is Q A. So that's 0.1 to the power four times 100, which is 0.0100 cubic centimeters per second. And then part E, they throw a whole bunch of changes at us. so we're going to replace the pressure difference with one and a half times what it was originally. I guess you can put an ‘A’ there. And then times pi times 0.1 times the original radius divided by eight times the original viscosity times half the length. And so this dividing by two here is the same as multiplying the whole fraction by two. Or you can multiply top and bottom by two, if you like, and with this two, we can cancel this two. And we are left with this factor two here. We have this factor one and a half, and then we have a factor 0.1 to the power four from here. And all of that gets multiplied by the stuff left over, which is Q A. So that's one and a half times two times 0.1 to the power four times a 100, which is 0.0300 cubic centimeters per second.