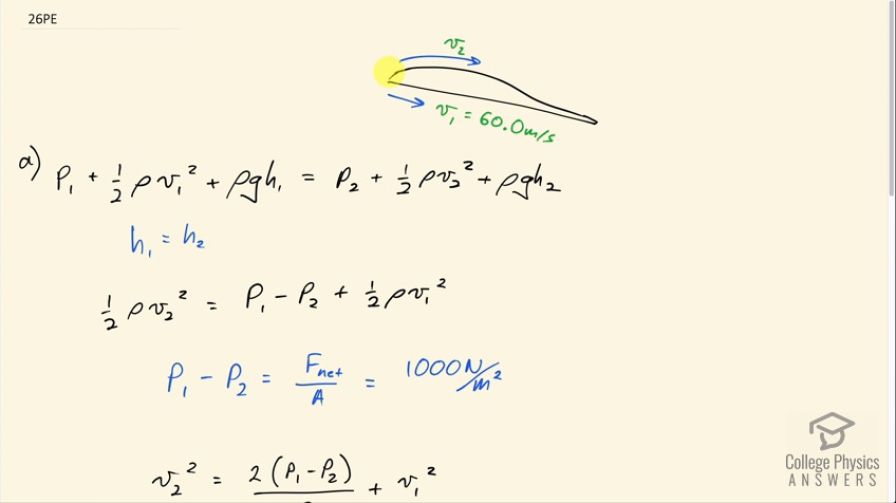
Question
A frequently quoted rule of thumb in aircraft design is that wings should produce about 1000 N of lift per square meter of wing. (The fact that a wing has a top and bottom surface does not double its area.) (a) At takeoff, an aircraft travels at 60.0 m/s, so that the air speed relative to the bottom of the wing is 60.0 m/s. Given the sea level density of air to be , how fast must it move over the upper surface to create the ideal lift? (b) How fast must air move over the upper surface at a cruising speed of 245 m/s and at an altitude where air density is one-fourth that at sea level? (Note that this is not all of the aircraft’s lift—some comes from the body of the plane, some from engine thrust, and so on. Furthermore, Bernoulli’s principle gives an approximate answer because flow over the wing creates turbulence.)
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 26 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An aircraft wing has air travelling at a speed of 60 meters per second on the bottom side and then over the top side is going to be traveling a bit faster because of this curve. And that's going to be V two and the question is, figure out what V two should be such that we get a force upwards in the wing lift force of 1000 newtons for every square meter of wing. So this is a unit of pressure, by the way. And so we're looking for the pressure difference in other words, of 1000 between the two sides of the wing. So, yes, there's Bernoulli's equation. And so the pressure on the bottom side of the wing, P one plus one half times the density of air times the speed of air there V one squared plus air density times g times the vertical height of this position, number one, equals the pressure on the topside of the wing P two plus one half air density times speed two squared plus rho g h two. Now these two terms are the same because the height difference is essentially negligible. This height is nearly the same as this height. There's not going to be much difference caused by that small height difference. The pressure, you know, air is not very dense. And so going up one meter from here to here isn't going to change the pressure any appreciable amount. So then we can rearrange this solving for one half rho V two squared by subtracting P two from both sides. We can write one half rho V two squared is P one minus P two plus one half rho V one squared. And we know what P one minus P two is. It's 1000 newtons per square metre, the pressure difference between the sides of the wing. And then we can solve for V two squared by multiplying both sides by two divided by density. So we divide this by two over density and multiply this by two over density. And so this second term becomes V one squared and the first term is two times the pressure difference divided by air density. So then take the square root of both sides to get an expression to the calculating V two. So the square root of two times the pressure difference divided by air density, plus the speed on the bottom side of the wing squared. So that’s square root of two times a 1000 newtons per square meter, divided by 1.29 kilograms per cubic meter, density of air at sea level times 60 meters per second squared, which is 71.8 meters per second. Now, when the plane goes to a higher altitude, a question is given the speed of 245 meters per second of air in the bottom of the wing and an air density one quarter what it is at sea level? So we're dividing by four here. What would the speed V two be? This works out to 257 meters per second.



