Question
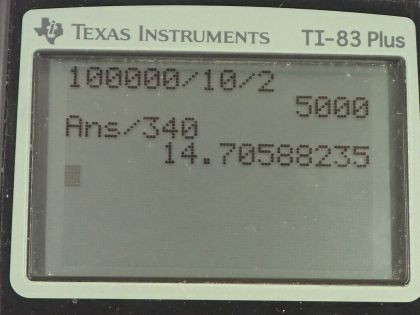
A mountain stream is 10.0 m wide and averages 2.00 m in depth. During the spring runoff, the flow in the stream reaches . (a) What is the average velocity of the stream under these conditions? (b) What is unreasonable about this velocity? (c) What is unreasonable or inconsistent about the premises?
Final Answer
- Mach # = 15. This is faster than the fastest fighter jet. It's much too fast for a stream.
- Either the stream is larger, or the volume flow rate is less.
Solution video
OpenStax College Physics, Chapter 12, Problem 16 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A mountain stream allegedly has a width of 10 meters and a depth of two meters and a volume rate of flow of 100,000 cubic meters per second. And our job in part A is to figure out what speed does the water have? So the volume rate of flow is the cross-sectional area of the stream multiplied by speed, and the area is going to be the streams width multiplied by its depth. We assume as a rectangular cross-section and we substitute that in for area w times d and then solve for V by dividing both sides by w d. So the speed, then is the volume rate of flow divided by width times depth. So it's 100,000 cubic meters per second divided by 10 meters times two meters, which is 5000 meters per second. That, to give some context, is Mach fifteen, if we divide that by the speed of sound 340 meters per second and this is faster than the fastest fighter jet, which is way too fast. So either the stream is much larger or the volume rate of flow is less.