Question
Hoover Dam on the Colorado River is the highest dam in the United States at 221 m, with an output of 1300 MW. The dam generates electricity with water taken from a depth of 150 m and an average flow rate of 650 m3/s650 \textrm{ m}^3\textrm{/s}. (a) Calculate the power in this flow. (b) What is the ratio of this power to the facility's average of 680 MW?
Question by OpenStax is licensed under CC BY 4.0
Final Answer
  1. 9.56×108 W9.56 \times 10^8 \textrm{ W}
  2. 1.411.41

Solution video

OpenStax College Physics, Chapter 12, Problem 25 (Problems & Exercises)

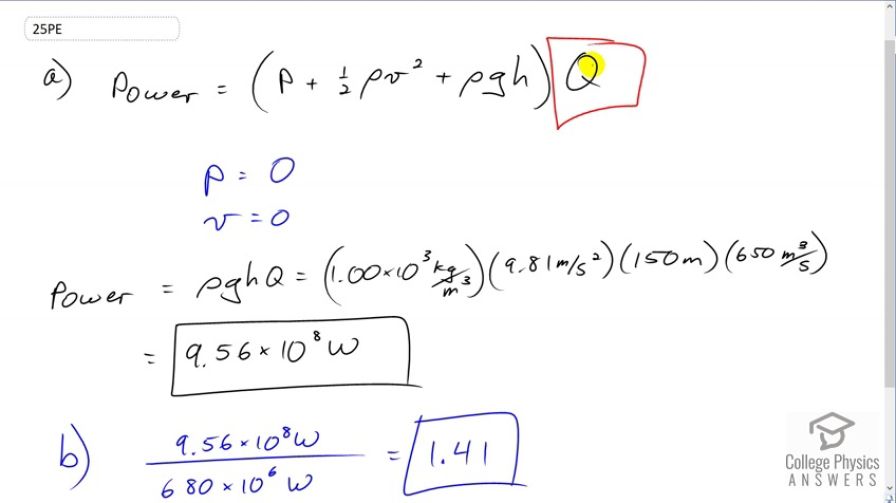
OpenStax College Physics, Chapter 12, Problem 25 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 12, Problem 25 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. Since the Bernoulli's Equation has units of Joules per cubic meter, that means if we multiply by the flow rate, which has units of cubic meters per second, we'll end up with Joules per second. Let me show that a bit better. So we have Joules per cubic meter from Bernoulli's Equation, so which of these terms has those units. And then, we're going to be multiplying by Q which is cubic meters per second. And the cubic meters will cancel leaving us with Joules per second which is abbreviated as watts. And so we're getting power by multiplying Bernoulli's Equation by the flow rate. And now, each of this terms in here represents differences between two different points. So, in the Hoover Dam we're looking for the difference in pressure between the surface of the water and where it exits the dam at a depth of 150 meters. Now, both of those locations will have the same atmospheric pressure. And so the pressure difference is zero. And we'll assume that where the water is entering, the hole in the dam, it's going to have some speed. But at the very entrance its speed will be zero. And so V is zero. And it's just this difference in height then that's going to result in the power. So the power is going to be the density of water times g times the height difference between the surface of the water and the hole in the dam times the volume flow rate. So that's one times ten to three kilograms per cubic meters times 9.81 meters per second squared times 150 meters depth times 650 cubic meters per second of volume flow rate giving us 9.56 times ten to the eight watts. And then we take that total possible power. This is the theoretical maximum power you could get from this water and divided by the actual power output in electricity of the dam. We got a ratio of 1.41 which actually shows fairly good efficiency. 1.0 would be perfect efficiency.

Comments

Shouldn't the height be 221m-150m?

Hi Emily,
Thank you for the question. You have the right idea: it's the different between the level of the water surface and the depth below that surface where the water is extracted that matters. However, I read the question as giving us a trivia fact for our own curiosity when it says the Hoover Dam height is 221m. That isn't the level of the water surface - if it was then the water would be spilling over the top of the dam and flooding the roadway on the top of the dam. The water surface level will always be maintained, by way of adjusting the intake gates, at a level below the top of the dam. With this in mind, it means we don't know the level of the water surface. It is not 221m. When the questions says "water taken from a depth of 150m" that means 150m below the water surface.
All the best,
Shaun