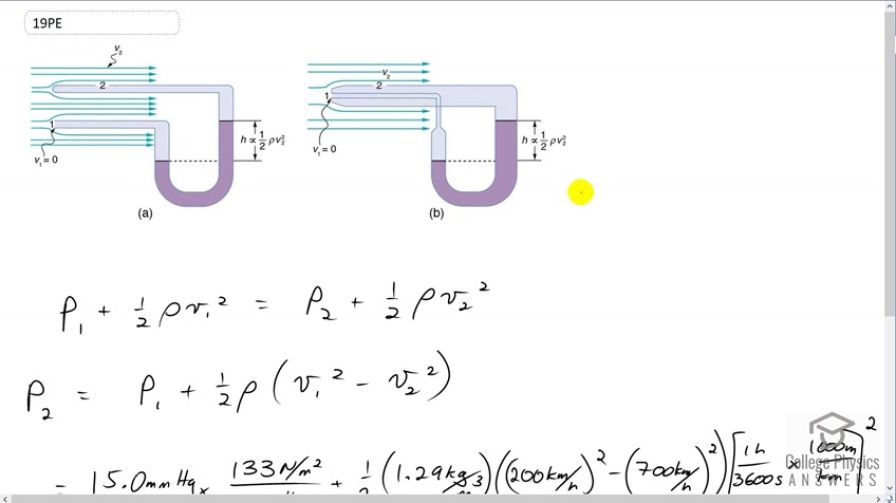
Question
If the pressure reading of your pitot tube is 15.0 mm Hg at a speed of 200 km/h, what will it be at 700 km/h at the same altitude?
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 19 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The way this question is worded where it says the pressure reading of U-Pattou Tube is blah blah blah is a bit confusing because pattou tubes don't measure pressure, instead they measure speed of the air going past these tubes here. And they measure that speed using pressure differences that arise in these two sides of this pipe here but this enough itself is not a measurement of the air pressure, it's only an indication of the air speed. So, what this question should be saying is what will the pressure be outside given a pattou tube telling you that the air speed is 700 kilometers per hour versus 200 kilometers per hour? So this is 15 millimeter of Mercury pressure reading did not come from the pattou tube, it's the 200 kilometers per hour that's what came from the pattou tube. So here's Bernoulli's principle and we're at constant altitude of course. So the plus rho gh terms disappeared from both sides because there is no difference in height. And so we have the pressure in the first case which is 15 millimeters in Mercury plus one half times the density of air times initial speed of 200 Kilometers in an hour equals the pressure in the second case which we want to find plus one half density of air times the speed in the second case squared. So we'll solve this for P2 by subtracting this term from both sides and then factor out the one half rho, common factor between these two terms. And we end up with this line. So the pressure is going to be the initial pressure plus one half times density times the difference in the squares of the velocities, so that's 15 millimeters of Mercury times 133 pascals per millimeter of Mercury plus one half times 1.29 Kilograms per cubic meter density of air times 200 kilometers per hours squared minus 700 kilometers per hour squared. And what I've done here is a bit unusual. Normally, I would convert this kilometers per hour here to meters per second, but I just felt like doing things a little differently. So, I'm taking the squares of the kilometer per hours and we have to multiply by the conversion factor here. And so that's one hour for 2,600 seconds times 1000 meters for every kilometer and we square that because we're converting this difference which will have units of kilometers squared per hours squared. If you didn't like this thing or this method, then just convert the 200 kilometers per hour into meters per second before substituting it and likewise with the 700. So we end with this milli pascals and we multiply it with one millimeter of Mercury for every 133 pascals and get negative 153 millimeters of Mercury will be the pressure when the air speed is 700 kilometers per hour.

