Question
(a) Calculate the momentum of a photon having a wavelength of (b) Find the velocity of an electron having the same momentum. (c) What is the kinetic energy of the electron, and how does it compare with that of the photon?
Final Answer
- 6.63\times 10^{-26} \textrm{ kg}\cdot \textrm{m/s}
- The energy of the photon is greater than the kinetic energy of the electron by a factor of 8240.
Solution video
OpenStax College Physics, Chapter 29, Problem 43 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
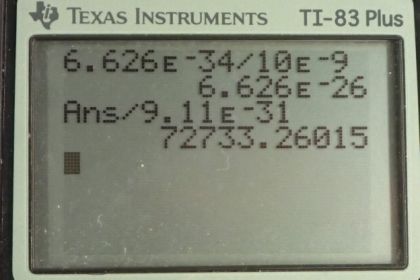
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. In part (a) of this question, let's find the momentum of a photon that has a wavelength of 10 nanometers. This is equation [29.22] and it is Planck's constant divided by the wavelength. So that's 6.626 times 10 to the minus 34 joule seconds divided by 10 times 10 to the minus 9 meters giving a momentum of 6.63 times 10 to the minus 26 kilograms meters per second. Then in part (b), we are asked to find the speed of an electron if it had that same momentum. And we are gonna assume the speed is non-relativistic in which case, we can use this formula for momentum; it is mass times velocity and we'll divide both sides by m and that gives a velocity of momentum divided by mass. So that's the answer for part (a) divided by the mass of an electron—9.11 times 10 to the minus 31 kilograms— giving a speed of 7.27 times 10 to the 4 meters per second and this is indeed non-relativistic because it's much less than something or 3 times 10 to the 8. In part (c), we are asked to compare the kinetic energies of the electron with the total energy of the photon. So the energy of the photon is hc over λ; that's equation [29.12], and we'll use this number—1240 elecron volt nanometers— in place of the product hc—that's [29.14], tells us that we can do that— and we'll divide by the wavelength, written in nanometers. So 1240 divided by 10 gives us energy in electron volts—124. So that's the energy of the photon. And then the kinetic energy of the electron is the next thing we are curious about and it is a non-relativistic velocity so we can use this formula— one-half mv squared— and that's one-half m times p over m because that's our expression for velocity. and we square that and this works out to p squared over 2m. This momentum we know from part (a) as an expression, h over λ, and this normally doesn't appear in a formula for an electron because this is momentum for a photon. But we are told that the electron has the same momentum as this photon. So we can plug h over λ in place of p and that gets squared and so we have an expression for the kinetic energy of the electron is 1 over 2 times the mass of the electron times Planck's constant over the wavelength and square that and then convert this into electron volts so that we can compare it to the energy for the photon, which is also expressed in units of electron volts. And this is 0.0150 electron volts. So finding the ratio of the energy of the photon—124 electron volts— to the kinetic energy of the electron— 0.0150 electon volts— is different by a factor of 8240. So the energy of the photon is greater than the kinetic energy of the electron by a factor of 8240.