Question
Red light having a wavelength of 700 nm is projected onto
magnesium metal to which electrons are bound by 3.68 eV.
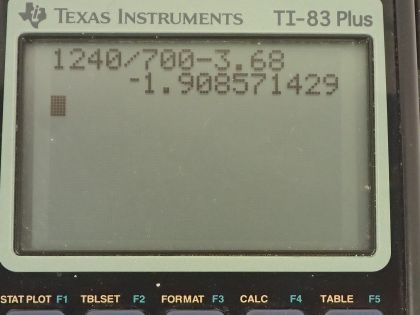
(a) Use to calculate the kinetic energy of e
the ejected electrons. (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?
Final Answer
- A negative kinetic energy is nonsense.
- The wavelength is too great, or it's false that an electron is ejected.
Solution video
OpenStax College Physics, Chapter 29, Problem 18 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Red light with a wavelength of 700 nanometers supposedly ejects an electron in magnesium metal that has a binding energy of 3.68 electron volts; in part (a), we are going to find the kinetic energy of this ejected electron: it's Planck's constant times the frequency of the photon minus the binding energy. Now we are given the wavelength of the photon so we have to rewrite frequency in terms of wavelength. The wave equation tells us that the speed of the wave is the wavelength times frequency and so we can divide both sides by λ to solve for f and then replace frequency with the speed of the wave divided by the wavelength. So the kinetic energy then is hc over λ minus binding energy and Planck's constant times speed of light can be written conveniently as 1240 electron volt nanometers when we divide by the wavelength, also in units of nanometers. So 1240 divided by 700 minus 3.68 electron volts gives us negative 1.91 electron volts. Now a negative kinetic energy is nonsense so that's our answer to part (b) here so there's something wrong with the information given to us in the question; either the wavelength is too large so in fact it should be smaller than 700 or it's false to say that an electron is actually ejected.