Question
(a) Calculate the number of photoelectrons per second ejected from a area of sodium metal by 500-nm EM radiation having an intensity of (the intensity of sunlight above the Earth’s atmosphere). (b) Given that the binding energy is 2.28 eV, what power is carried away by the electrons? (c) The electrons carry away less power than brought in by the photons. Where does the other power go? How can it be recovered?
Final Answer
- The remaining energy goes into the sodium metal in the form of mass equal to the binding energy. If the electron was ejected with less than the maximum kinetic energy some the energy would also be in the form of, probably, heat or an energetically excited state of sodium. The energy transformed into mass (which is the binding energy, in other words) will be released again as a photon or heat when the electron is recaptured by the sodium ion.
Solution video
OpenStax College Physics, Chapter 29, Problem 17 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
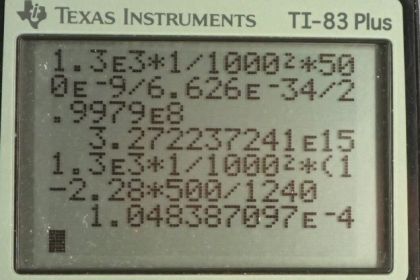
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A one square meter piece of sodium is illuminated by photons with a wavelength of 500 nanometers and an intensity of 1.30 kilowatts per square meter. And the question is how many photoelectrons are ejected per second? Well, we know that one photoelectron is ejected by each photon and so we need to account the number of photons in a beam with this intensity on this area. So intensity is defined as the power per area and power is the total energy of the photons per second or per time. And the total energy of all these photons is the number of photons multiplied by the energy per photon and so we can replace a few things in this intensity formula; we'll write intensity is the total energy which is number of photons multiplied by the energy per photon over time times area. And then we can solve for this n over t because this is the number of photons per second which will equal the number of electrons ejected per second. And we'll multiply both sides by A over energy of a photon on both sides. And we have that the number of photons per time is the intensity times the area divided by the energy per photon. And the energy per photon is Planck's constant multiplied by the frequency but we don't know the frequency, we are given the wavelength instead so from the wave equation which says that the speed of a wave equals frequency times wavelength, we can solve for f and say that it's c divided by λ and so we'll replace f with c over λ here. And then we are dividing by this and so let's multiply by its reciprocal in order to avoid dividing a fraction by a fraction because that gets messy so we'll multiply by λ over hc here. And that means the number of photons or therefore, the number of electrons ejected per time is gonna be 1.30 times 10 to the 3 joules per second per meter squared— that's the intensity of kilowatts per square meter but instead of writing watts, I wrote joules per second— and multiply that by 1 square millimeter converted into square meters by multiplying by 1 meter for every 1000 millimeters, twice, and then multiply by the wavelength— 500 times 10 to the minus 9 meters— and divide that by Planck's constant times the speed of light which gives 3.27 times 10 to the 15 photoelectrons per second; photoelectrons is just a fancy way of saying electrons that were ejected by a photon, you could just write the word 'electrons' and that might be more clear. There. So that's the rate at which electrons are ejected from the sodium. And part (b) asks us given the certain binding energy for sodium of 2.2 electron volts, what power is carried away by the electrons? So the electrons will have some kinetic energy because this energy of the photons exceeds the binding energy and so that difference is gonna be kinetic energy of the electrons. And so what is the rate at which that energy is being carried away, that's the question here. And this power is a different power than this one here in part (a); this is the total energy of the photons per time and that is not the power we are concerned with in part (b) and so that's why I gave it this apostrophe, this prime symbol to distinguish it. So this is the power contained in the kinetic energy of the ejected electrons. So the kinetic energy of an ejected electron is the energy of the photon minus the binding energy and we'll replace f with c over λ and so we have kinetic energy of the electron is Planck's constant times speed of light over the wavelength minus binding energy and we are told that binding energy is 2.28 electron volts for sodium and the power carried away by the electrons is the kinetic energy per electron multiplied by the number of electrons so that's the total energy carried by the electrons per time. But this n over t; we have an expression for it already which is up here—IAλ over hc and so that's where this comes from— IAλ over hc is n over t. And the kinetic energy we have an expression for that here and so I have written hc over λ minus binding energy in place of kinetic energy of the electrons. And so I simplified it a little bit and multiplied into the brackets by this factor outside and we multiplied the λ over hc into the bracket anyhow. And so we have IA outside the brackets times 1 because λ over hc times hc over λ is 1 minus the binding energy times λ over hc. So the power carried away by the electrons then is the intensity—1.30 times 10 to the 3 watts per square meter— times the area converted into meters and then times by 1 minus 2.28 electron volts—binding energy— times the wavelength of 500 nanometers and this gives units of electron volt nanometers in the numerator and so for Planck's constant times speed of light in the denominator, we choose 1240 electron volt nanometers as our figure, which is given to us as [29.12] in the textbook; this is just for convenience and then these units will cancel and we end up with 0.105 milliwatts of power carried away by the electrons. So what about the rest of the energy of the photons, where does that energy go? So this is the rate at which energy is carried away in the form of kinetic energy by the electrons and there's still lots more energy involved which has been absorbed into the sodium and it gets absorbed in the form of mass so it increases the mass of the sodium metal, just a tiny bit, an amount equal to the binding energy. And so let me read this and make sure I can explain it properly; if the electron was ejected with less than the maximum kinetic energy and there's probably some heat as well, I suppose, but for the most part, what's important to understand is that the binding energy results from a change in mass of the substance so by having an electron ejected, the sodium metal absorbs some of the photon energy and by doing so, increased its mass as a result and then if the electron is rebound to the sodium ion that energy will then be released again as a result of the sodium losing some mass and it will release an amount of energy equal to the binding energy if the electron is recaptured.