OpenStax College Physics, Chapter 29, Problem 60 (Problems & Exercises)

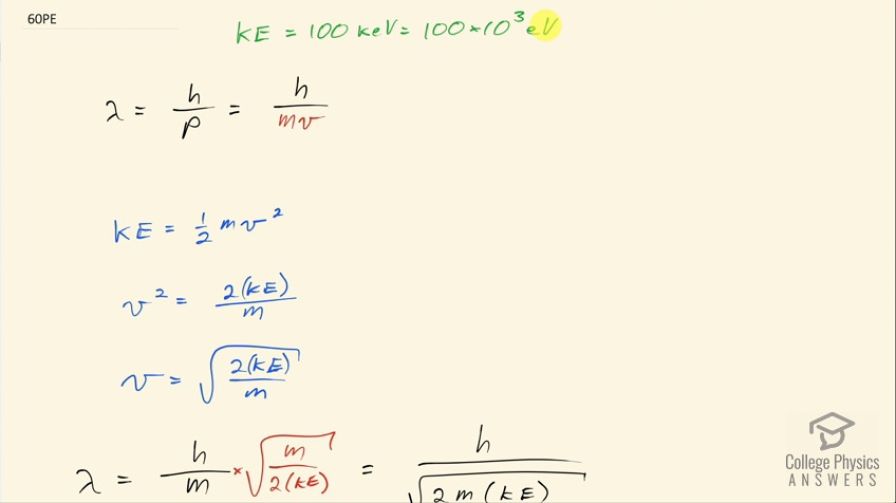
This is College Physics Answers with Shaun Dychko. An electron has a kinetic energy of 100 kiloelectron volts, which is 100 times 10 to the 3 electron volts and the question is assuming non-relativistic speed, what is this electron's wavelength? So its wavelength will be Planck's constant divided by its momentum and since we are told that this is a non-relativistic speed, we can substitute mv in place of p otherwise we would need a γ— Lorentz factor its called— here as well but we don't need that since the speed is non-relativistic. We need to figure out the electron's speed in order to substitute for this unknown v here in this formula in order to calculate the wavelength so we'll use the kinetic energy to figure out the speed; kinetic energy is one-half mass times velocity squared and we can solve for v squared by multiplying both sides by 2 over m and so we have v squared then is 2 times kinetic energy divided by mass after you switch the sides around and then take the square root of both sides to solve for v and then we are going to substitute this in place of v in our formula for λ. Now since we are dividing by v and this v is a fraction, I prefer to multiply by the reciprocal of this denominator because I think it's messy to have a fraction within a fraction so I am going to take the reciprocal of this, which is to say I am going to flip it over and multiply this whole fraction by this flipped over. So we have h over m then multiplied by square root m over 2 times kinetic energy and the square root m in the numerator and this m to the power of 1 in the denominator there results in m to the one-half or square root m in the denominator and so this simplifies to h over square root 2m times kinetic energy. So we have Planck's constant here divided by the square root of 2 times the mass of an electron times 100 times 10 to the 3 electron volts converted into joules because we have mks units in our other factors here and so we multiply by 1.602 times 10 to the minus 19 joules per electron volt and this works out to 3.88 picometers and the prefix 'pico' means multiply by 10 to the minus 12.