Question
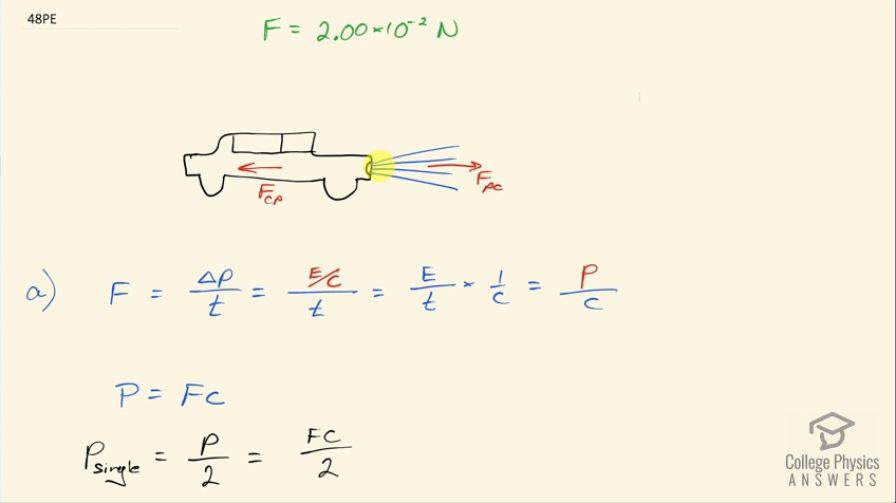
A car feels a small force due to the light it sends out from its headlights, equal to the momentum of the light divided by the time in which it is emitted. (a) Calculate the power of each headlight, if they exert a total force of backward on the car. (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?
Final Answer
- This is too large. It is about 5% the power output of a typical power plant (600 MW)
- The light would not exert such a large force.
Solution video
OpenStax College Physics, Chapter 29, Problem 48 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This car is experiencing a force backwards due to the force on the photons forwards to shoot the photons out of the headlights and while this idea is true, the magnitude of this force is far too big as we'll see soon. So... we have the force on the photons due to the car to the right and then there's a Newton's Third Law counterpart, which is the force on the car due to those same photons going back and force can be written as mass times acceleration— that's the typical way to get introduced to the idea of net force— but this can also be written as mass times velocity over time and this numerator is momentum— mass times velocity— so this can instead be written as momentum over time; the rate of change of momentum in other words is the force and momentum for a photon is the photon energy divided by speed of light and so this is energy over time multiplied by 1 over c that's kind of a strange way of writing this fraction but I am doing that in order to show that this is power and so this P here is a different P than this one by the way— that's a bit confusing... sorry but let's make it capital P... that's power not momentum— so this is power divided by speed of light and power is F times c, in other words, if you multiply this by c and then the other side by c so the power is the force times the speed of light and we know what the force is and we can substitute in speed of light as well but we want the power of a single headlight and so this is the total power here because we have the total force and so we'll divide that by 2 because there's two headlights so we have Fc over 2 is going to be our answer and that's going to be 2.00 times 10 to the minus 2 newtons— total force— times speed of light divided by 2 and that is 3.0 megawatts. This power of the headlights supposedly is way too large... it's about 5 percent the typical power output of a coal-fired power plant, which is about 600 megawatts. So it's too large and the light would not exert such a large force that's a false assumption to think that these photons from these headlights are exerting a force of 2.00 times 10 to the minus 2 newtons.
