Question
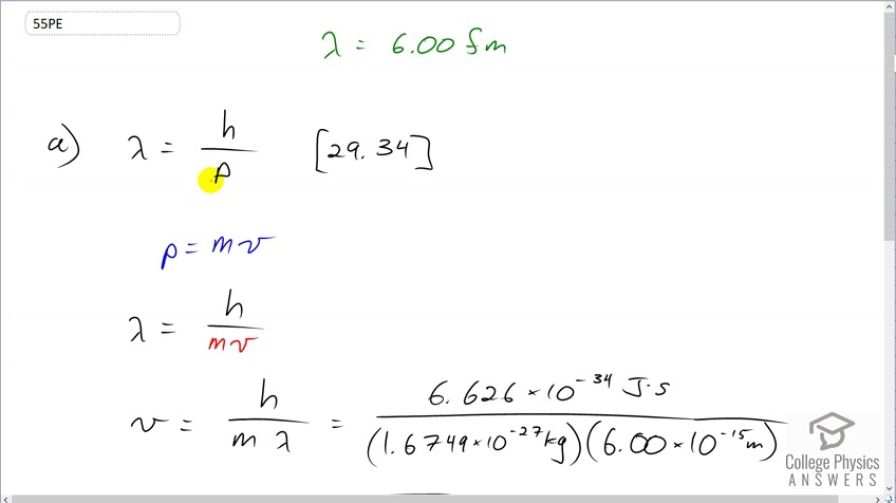
(a) Find the velocity of a neutron that has a 6.00-fm wavelength (about the size of a nucleus). Assume the neutron is nonrelativistic. (b) What is the neutron’s kinetic energy in MeV?
Final Answer
Solution video
OpenStax College Physics, Chapter 29, Problem 55 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to find the velocity of a neutron that has a wavelength of 6 femtometers. So the wavelength is Planck's constant divided by momentum and we can substitute mv in place of momentum. So the wavelength is Planck's constant over mass times velocity. And we'll solve this for v by multiplying both sides by v over λ. And so we have the velocity is Planck's constant over the mass of the neutron times the wavelength. So that's Planck's constant—expressed in units of joule seconds— divided by mass of a neutron times its wavelength, which is 6 times 10 to the minus 15 meters which is a velocity of 6.59 times 10 to the 7 meters per second. And part (b) says find the kinetic energy of this neutron in units of megaelectron volts. So we'll go one-half mass times velocity squared. So that's one-half mass of the neutron times velocity that we found in part (a), written with a few more decimals here, and then we square that and then we get units of joules here because joules is an mks unit— meters, kilograms and seconds. And so we need to change that into electron volts by multiplying by 1 electron volt for every 1.602 times 10 to the minus 19 joules and then convert that further to megaelectron volts by multiplying by 1 megaelectron volt for every 10 to the 6 electron volts. This gives 22.7 megaelectron volts of kinetic energy.
