OpenStax College Physics, Chapter 29, Problem 33 (Problems & Exercises)

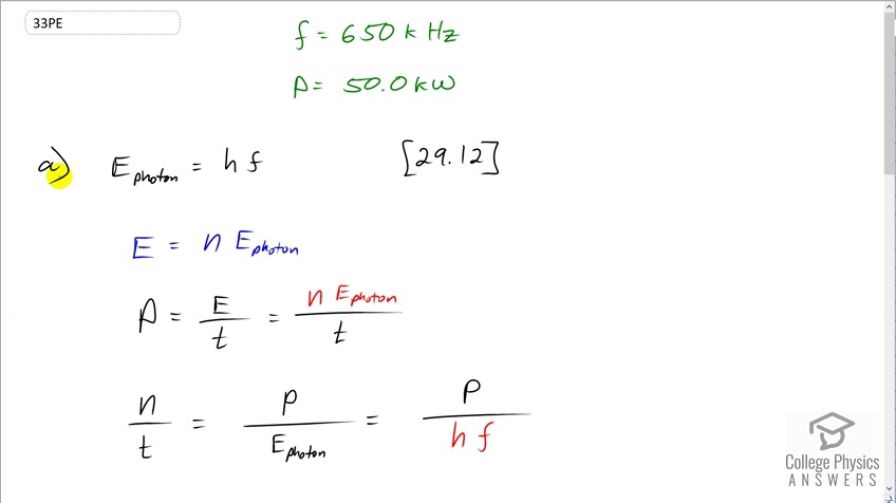
This is College Physics Answers with Shaun Dychko. A radio station with a power of 50 kilowatts transmits a frequency of 650 kilohertz. In part (a), we want to figure out how many photons are emitted per second and then in part (b), we'll figure out how many photons per second pass through a square meter that is positioned 100 kilometers away from the transmitting tower? So to figure out the number of photons per second, we'll take this power output that we know which is the total energy emitted divided by time and this total energy is going to be the number of photons multiplied by the energy per photon. And this energy per photon we know from this formula [29.12], which is Planck's constant times the frequency. And this n over t—the number of photons per time— is what we want to know in part (a) and so we'll solve for that by dividing both sides by the energy of a single photon. And so n over t—number of photons per time— is a power divided by the energy of a single photon, which we substitute with hf. And so that's 50 times 10 to the 3 watts of power divided by Planck's constant times 650 times 10 to the 3 hertz and this gives us 1.16 times 10 to the 32 photons per second and we know it's per second because this watts can be thought of as joules per second and these joules will cancel and these seconds will cancel with this hertz because hertz can be written as 1 over seconds; hertz is an abbreviation for reciprocal seconds, and these seconds cancel leaving us with 1 over seconds and the units in the numerator are photons because we are counting photons seeing as this is the energy per photon. OK. So part (b) says consider a position 100 kilometers away, what would be the number of photons traveling through it per second if there's a 1 square meter panel located this distance away? So this is a type of flux, flux being the rate at which something passes through an area. So area is going to be the surface area of a sphere because this energy transmitted or the photons transmitted by this tower are going to be distributed evenly over a sphere and the sphere has a area of 4π times radius squared and the radius is this r given to us— 100 kilometers. And so the flux then is the rate of photons being transmitted, which we figured out in part (a), divided by the area of this sphere here. So this is 1.16093 times 10 to the 32 photons every second divided by 4π times 100 times 10 to the 3 meters squared which gives 9.24 times 10 to the 20, I should say, photons per second per square meter. So 1 square meter would have this many photons passing through it every second.