OpenStax College Physics, Chapter 29, Problem 70 (Problems & Exercises)

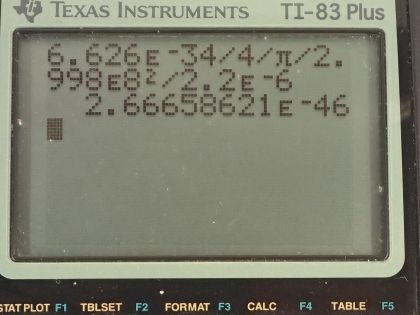
This is College Physics Answers with Shaun Dychko. We need to calculate the uncertainty in the mass of a muon based on the uncertainty in its lifetime. So we will take the uncertainty in its lifetime to be the muon's lifetime, which I looked up in Wikipedia— it's 2.2 microseconds— and the uncertainty in its mass energy multiplied by the uncertainty in this time measurement is greater than or equal to Planck's constant over 4π and we can substitute mc squared in place of E— we assume this particle is at rest— and its energy is its mass times its speed of light squared. Now the uncertainty in this product is going to be attributable just to the uncertainty in the mass since we'll take the uncertainty in the speed of light to be you know, zero basically... it's one of these fundamental constants. So we can divide both sides by Δt times c squared and so we have the uncertainty in mass then is Planck's constant over 4π times speed of light squared times the uncertainty in time. So that's 6.626 times 10 to the minus 34 joule seconds divided by 4π times the speed of light squared times the lifetime of the muon and this works out to an uncertainty in mass of more than or equal to 2.7 times 10 to the minus 46 kilograms.