Question
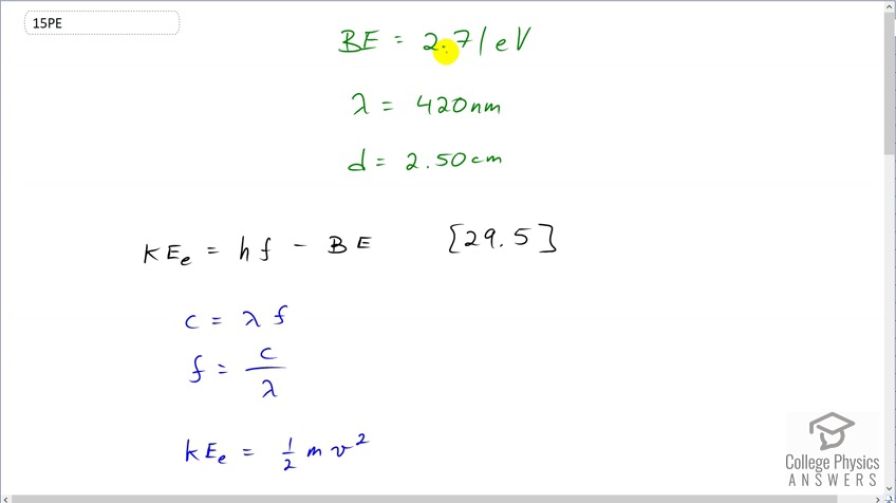
Photoelectrons from a material with a binding energy of 2.71 eV are ejected by 420-nm photons. Once ejected, how long does it take these electrons to travel 2.50 cm to a detection device?
Final Answer
Solution video
OpenStax College Physics, Chapter 29, Problem 15 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Some photons with wavelength, 420 nanometers, eject some electrons from a material that has a binding energy of 2.71 electon volts. And these electrons will have some kinetic energy, given by this formula, and we want to know how long it will take for those electrons to travel this distance of two and a half centimeters. So the maximum kinetic energy the electrons will have will be Planck's constant times the frequency of the photons minus the binding energy of the material and we'll replace frequency with an expression in terms of lambda by knowing that the wave equation is wavelength multiplied by frequency and we'll divide both sides by the wavelength and we get frequency is speed of light divided by wavelength. And then we'll replace f with that here. So instead of f, I have written c over λ. We also know the kinetic energy, assuming this electron is non-relativistic, is going to be one-half the electron mass times its velocity squared so we can substitute that here. And so what we are trying to do is figure out what is the speed of the electron after it's ejected and then knowing the distance it has traveled, we'll figure out what time it takes to travel that distance. So we wanna solve for v here so let's multiply both sides by 2 over m and we get v squared is 2 over m times Planck's constant times speed of light over wavelength minus binding energy and then take the square root of both sides and we have an expression for v. One of our Kinematics formula says that the distance traveled is the speed times time and we'll divide both sides by the speed to solve for t. So t is d over v and we just created a huge expression for v that we'll substitute in place here, which I have done in red. So the time is going to be the distance the electron has to travel— two and a half centimeters— divided by the square root of 2 divided by electron mass times Planck's constant times speed of light over the wavelength of the photon, that ejected the electron, minus binding energy. So that's 2.50 times 10 to the minus 2 meters divided by square root of 2 over 9.11 times 10 to the minus 31 kilograms— mass of an electron— times Planck's constant times speed of light divided by the wavelength in meters; that's 420 times 10 to the minus 9 meters minus the binding energy, converted into joules, because this term is in joules and so this term also has to be in joules and we want this whole thing to be in units of joules because joules is an mks unit—a meters, kilograms, seconds unit— which will match with these kilograms and those meters and so our final answer will end up having seconds, as we expect. So that's 2.71 electon volts—binding energy— multiplied by 1.602 times 10 to the minus 19 joules for every electron volt and we end up with 85.6 nanoseconds, is the time the electron will take to travel two and a half centimeters.
